Note that in all of the joining methods described above, the beams are not diminished in cross-section, so full shear and bending strength is maintained.
1 cannot over-emphasize the importance of maintaining a good standard of “build quality.” One member should bear flat on another, without wobbling. The end of a beam should bear at least four inches on the supporting post or beam below, if possible. Timbers should be vertically plumb and horizontally level. The design should assure that the line of thrust is always transferred directly in compression from one member to another.
By paying attention to the build quality, you enlist a great ally, which is the force of gravity. Gravity is very precise: it always works exactly vertically (which enables a bubble-level to function properly, by the way). And it is reliable; that is to say, it is working for you every morning when you wake up, and through the night as well.
Gravity and its close relative inertia are very important parts of all heavy timber-framed structures. Earth-roofed structures help even more in this regard. Our roof at Earthwood weighs between 6о and 120 tons, depending on moisture and snow loads. Even at the low end (dry, no snow), the 6o-ton load has a tremendous inertia. The seven load-bearing eight-by-eight posts downstairs are not even pinned to the floor; with at least three tons on each post, they aren’t going anywhere. (I know, three times seven tons is only 21 tons. The external walls and central masonry column support the rest.) Now, I don’t advise not pinning the posts to the floor — “Do as I say, not as I do!” — but I just thought you might find our experience interesting.
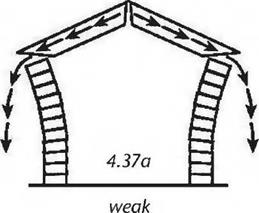
Gravity can work against you, too. You have probably seen lots of diagrams like Fig. 4.37. These drawings depict freestanding cordwood walls, which are strong on compression and weak on tension. Using cordwood exaggerates the effect of the stresses a bit, but helps make the point. The same sorts of compression and tension forces are at play in a post-and-beam wall. They may be less obvious, but they need to be attended to for the same reasons.
In Fig. 4.37a, the roof load wants to follow gravity’s path, but the angle of the rigid rafters transfers the downward thrust to an outward thrust on the walls, and the building falls down. This would be an excellent example of egregiously bad build quality. In Fig. 4.37b, the tie beam has a tensile strength sufficient to offset
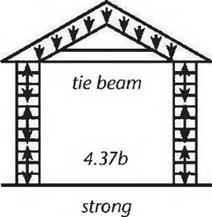
the outward thrust. Another way of thinking about it is that the tie beam turns the roof structure into a giant rigid triangle, a triangle with — and this is important — a flat bottom. Gravity’s downward thrust is carried straight down onto the vertical walls. This is how trusses work.
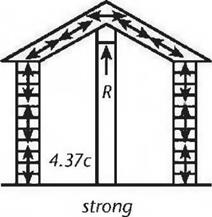
In Fig. 4.37c, rafters are well-tied to a ridge beam, which, in turn, is supported by posts. If the tops of the rafters can’t go down — and they can’t because of the reactionary load “R” provided by the posts — then they cannot put an outward thrust on the walls.
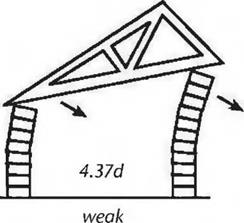
The importance of level and plumb is illustrated in Fig. 4.3yd. Here, we have a nice rigid triangular truss, but the bottom chord of the truss, which is inclined, alters the vertical line of thrust. This resultant vector of force places unacceptable stresses on the walls, as shown. Working with gravity, and not against it, is always a good idea. In this example, if the walls were the same height, and the bottom chord was horizontal, the line of thrust would be straight down onto the walls, which — being strong on compression — would then provide the required reactionary load.
Build quality, gravity, and inertia can be important allies… or deadly enemies.
