Getting around problems and obstacles is part of the plumbing and pipe fitting trades. Few jobs run without problems or obstacles. As any experienced piping contractor knows, there are always some obstructions in the preferred path of piping. Many times the obstacles are ductwork, but they can involve electrical work, beams, walls, and other objects are that not easily relocated. This means that the pipes must be rerouted. This section is going to deal with the mathematics required to compensate for immovable objects.
Let me set the stage for a graphic example of getting around an overhead obstruction. Assume that you are bringing a pipe up and out of a concrete floor in a basement. There is a window directly above the pipe that you must offset around. The window was an afterthought. Having the pipe under the window was not a mistake in the groundworks rough-in. However, it is your job to move the pipe, without breaking up the floor, to get around the window.
in many cases, you might just cut the pipe off close to the floor, stick a 45° fitting on it, and bring a piece of pipe over to another 45° fitting. This is usually enough, but suppose you have a very tight space to work with and must make an extremely accurate measurement. Do you know how to do it? Imagine a situation where an engineer has indicated an exact location for the relocated riser. Can you hit the spot accurately? Do you know what type of formula to use in order to comply with the job requirements? if not, consider the following information as your ticket to success.
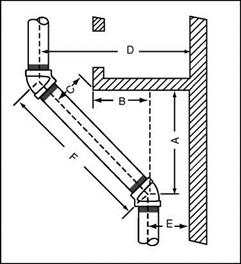
Our formula will involve three symbols. The first symbol will be an A, and it will be representative of the distance from the center of your 45° fitting to bottom edge of the window. The distance from the center of the rising pipe to the outside edge of the window will be known by the letter B. We will use the letter C to indicate the distance from the center of the Travel piece of
|
FIGURE 2.7 ■ Pipe offsets around obstructions. |
pipe from the edge of the window. Using E to indicate the distance of the center of the rising pipe from the right edge of the window and D to indicate the center of the offset rising pipe from the right edge of the window, we can use the formula. Let’s see how it works.
To find the distance from the bottom of the window to the starting point of the offset, you would take the distance from the center of the riser to the left edge of the window (B) and add the distance from the corner of the left window edge to the center of the pipe (C) times 1.414. The formula would look like this: A = B + C X 1.414. Refer to Fig. 2.7 for an example. Now let’s put measurement numbers into the formula.
Assume that you want to find A. Further assume that B is equal to one foot and that C is equal to six inches. The numerical formula would be like this: A = 12 inches (B) + 6 inches (C) X 1.414 = 12 inches + 8V2 inches = 20V2 inches. This would prove that the upper 45° fitting would be 20V2 inches from the edge of the right edge of the window. As you can see, the actual procedure is not as difficult as the intimidation of using formulas might imply.
