Cumulative hazard function and average failure rate

Similar to the cumulative distribution function (CDF), the cumulative hazard function can be obtained from integrating the instantaneous hazard function h(t) over time as

H(t) = f h(t) dt

J0

Referring to Eq. (5.3), the hazard function can be written as

1 d [pf (t)] 1 d [ps(t)]

Ps (t) dt ps(t) dt

Multiplying dt on both sides of Eq. (5.6) and integrating them over time yields

under the initial condition of ps(0) = 1.

Unlike the CDF, interpretation of the cumulative hazard function is not sim­ple and intuitive. However, Eq. (5.7) shows that the cumulative hazard function is equal to ln[1/ps(t)]. This identity relationship is especially useful in the sta­tistical analysis of reliability data because the plot of the sample estimation
of 1/ps(t) versus time on semi-log paper reveals the behavior of the cumula­tive hazard function. Then the slope of ln[1/ps(t)] yields directly the hazard function hit). Numerical examples showing the analysis of reliability data can be found elsewhere (O’Connor, 1981, pp. 58-87; Tobias and Trindade, 1995, pp. 135-160).

Since the hazard function h(t) varies over time, it is sometimes practical to use a single average value that is representative of the failure rate over a time interval of interest. The averaged failure rate (AFR) in the time interval [t1, t2] can be defined as

/t? h(t)dt t2 — t1	ln[ps(t1)] — ln[ps(t2)] t2 — t1
h (t2) — h (tp t2 — t1
AFR(t1, t2)	(5.8)

Therefore, the averaged failure rate of a component or system from the begin­ning over a time period (0, t] can be computed as

AFR(0, t) = —lj[p-lt)] (5.9)

The failure rate, in general, has the conventional unit of number of failures per unit time. For a component with a high reliability, the failure rate will be too small for the conventional unit to be appropriate. Therefore, the scale frequently used for the failure rate is the percent per thousand hours (%K) (Ramakumar, 1993; Tobias and Trindade, 1995). One percent per thousand hours means an expected rate of one failure for each 100 units operating 1000 hours. Another scale for even higher-reliability components is parts per million per thousand hours (PPM/K), which means the expected number of failures out of one million components operating for 1000 hours. The PPM/K is also called the failures in time (FIT). If the failure rate h(t) has the scale of number of failures per hour, it is related to the %K and PPM/K as follows:

1%K = 105 x h(t) 1 PPM/K = 1 FIT = 108 x h(t)

Example 5.1 Consider a pump unit that has an exponential failure density as

ft(t) = ke~lt for t > 0, к > 0

in which к is the number of failures per unit time. The reliability of the pump in time period (0, t], according to Eq. (5.1), is

/

TO

ke-kt dt = e-kt

as shown in Table 5.1. The failure rate for the pump, according to Eq. (5.3), is

ft (t)

ps(t)

which is a constant. Since the instantaneous failure rate is a constant, the averaged failure rate for any time interval of interest also is a constant.

Example 5.2 Assume that the TTF has a normal distribution with the mean /xt and standard deviation at. Develop curves for the failure density function, reliability, and failure rate.

Solution For generality, it is easier to work on the standardized scale by which the random time to failure T is transformed according to Z = (T — /xt)/at. In the stan­dardized normal scale, the following table can be constructed easily:

(1) z	(2) Ф (z)	(3) Pf (z) = Ф (z)	(4) Ps(z)	(5) h(z)	-t? S’ ^ II <
—3.5	0.0009	0.0002	0.9998	0.0009	0.0000018
—3.0	0.0044	0.0014	0.9986	0.0044	0.0000088
—2.5	0.0175	0.0062	0.9938	0.0176	0.0000352
—2.0	0.0540	0.0228	0.9772	0.0553	0.0001106
—1.5	0.1295	0.0668	0.9332	0.1388	0.0002776
—1.0	0.2420	0.1587	0.8413	0.2877	0.0005754
—0.5	0.3521	0.3085	0.6915	0.5092	0.0010184
0.0	0.3989	0.5000	0.5000	0.7978	0.0015956
0.5	0.3521	0.6915	0.3085	1.1413	0.0022826
1.0	0.2420	0.8413	0.1587	1.5249	0.0030498
1.5	0.1295	0.9332	0.0668	1.9386	0.0038772
2.0	0.0540	0.9772	0.0228	2.3684	0.0047368
2.5	0.0175	0.9938	0.0062	2.8226	0.0056452
3.0	0.0044	0.9986	0.0014	3.1429	0.0062858
3.5	0.0009	0.9998	0.0002	4.5000	0.0090000

NOTE : at = 500 hours; h(t) has a unit of failures/h, t = t + atz.

Column (2) is simply the ordinate of the standard normal PDF computed by Eq. (2.59). Column (3) for the unreliability is the standard normal CDF, which can be obtained from Table 2.2 or computed by Eq. (2.63). Subtracting the unreliability in column (3) from one yields the reliability in column (4). Then failure rate h(z) in column (5) is obtained by dividing column (2) by column (4) according to Eq. (5.3).

Note that the failure rate of the normal time to failure h(t) = ft(t)/ps(t) is what the problem is after rather than h(z). According to the transformation of variables, the following relationship holds:

ft (t) = Ф (z)dz/dt = ф (z)/at

Since ps(t) = 1 — Ф^), the functional relationship between h(t) and h(z) canbederived as

h(t) = h( z)/fft

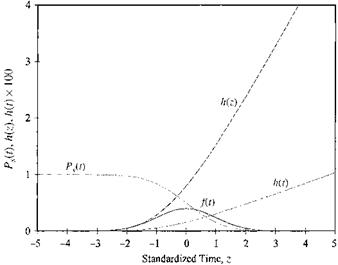
Column (5) of the table for h(t) is obtained by assuming that at = 500 hours. The relationships between the failure density function, reliability, and failure rate for the standardized and the original normal TTF are shown in Fig. 5.7. As can be seen, the failure rate for a normally distributed TTF increases monotonically as the system ages. Kapur and Lamberson (1977) showed that the failure-rate function associated with a normal TTF is a convex function of time. Owing to the monotonically increasing characteristics of the failure rate with time for a normally distributed TTF, it can be used to describe the system behavior during the wear-out period.

Figure 5.7 Reliability [ps(t)], failure rate [h(t)], failure density func­tion [ ft (t)] for a process/component the TTF of which follows a normal distribution as in Example 5.2.