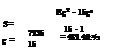A dam to protect Tiryns

Posted by on 16/ 11/ 15

A catastrophe strikes the city of Tiryns toward the end of the Mycenaen civilization. The city is located on an alluvial plain about one kilometer from the sea; the palace is 24 m above sea level, on a limestone hill. The city itself is at the foot of the palace, to the east and south. The watercourse along which the city is located, the Lakissa, leaves the mountains on a steep slope of nearly 15 m/km as dictated by the local topography.

Levees normally protect the city from the caprices of the river. But in about 1200 BC, at essentially the same time that the nearby city of Mycenae and its palaces were destroyed and burned, an exceptional event occurred. The Lakissa River left its bed, flowed to the north across the lower city, and covered most of the city with a thick layer of sediments, as much as 4 m deep in places. Only the palace on its hill in the southern part of the city was spared the effects of the deluge. This event could have been caused simply by an exceptional flood, or more likely by a major earthquake, the same one that destroyed Mycenae. Such a quake could have caused the collapse of the riverbanks causing a wave of debris-laden water rushing down the steep slope of the riverbed.[154]

A dam to protect Tiryns

But this event did not mark the end of the Mycenaens. To protect the city from the Lakissa, they built a dam 3.5 km upstream to divert waters of the Lakissa into a 1.5 km canal for conveyance to another watercourse, the Manessi, that flows more to the south (Figures 4.12, 4.13 and 4.14). This 10-m high dam, extending 57 m and 103 m across the left and right banks, respectively, is built of earth fill between two walls of the same type of cyclopean masonry[155] that is used in other Mycenaen works. The lower city is rebuilt on top of the sediments that buried the old city, eventually occupying about the same overall area as before (see inset in Figure 4.12).

Подпись: Figure 4.13 The Kofini dam for protection of the city of Tiryns against floods of the Lakissa (present-day situation) - Zangger, 1994.
A dam to protect Tiryns

A dam to protect TirynsFigure 4.14 Lakissa diversion canal, looking from the top of the Kofini dam (photo by the author)

This account illustrates the kinds of natural catastrophes that, in the troubled climate of the years around 1200 BC, may have contributed as much to the destruction of the palaces as did the Dorian invaders. Nonetheless, the Mycenaen civilization was still strong enough to implement the hydraulic works that returned the city of Tiryns to safe­ty. A century later this civilization of Nestor, Menelaus and Agamemnon, warrior-
builder descendents of the Minoans, does finally succumb to the overwhelming pressure of the Dorians, leaving only legends behind.

Interior trim

Posted by on 16/ 11/ 15

Interior trim

might see on a Victorian-style house. Because I want the trim to look good,

I use hot-dipped galvanized, stainless – steel, or aluminum finish nails when installing exterior casing so that the nails won’t rust and stain the wood.

Interior casing goes around interior doors and windows and hides the gap between the drywall and doorjambs or window frame (see the drawing above). The joint where the side piece meets the casing at the top is usually a 45° miter.

Flat, rectangular casings that are wider and more affected by shrinking/swelling cycles are often cut square and butted together. Use finish nails to attach cas­ing so they can be set below the surface and covered with wood putty.

The stool (sometimes called a sill) is the flat piece of trim installed at the bottom of a window (a perfect place to set flowers). Some windows have a stool built in as part of the frame, but more often than not the stool needs to be in-

stalled separately. A piece of trim called an apron covers the joint between the stool and the drywall below the window.

Baseboard runs horizontally and covers the joint between the wall and the floor. It is usually joined at outside corners with a 45° miter joint and is nailed to the wall with finish nails after the finish floor is installed. Interior corners can be joined with a 45° miter or with a coped joint (where one piece of trim is cut to match the profile of the mating piece).

Even though there are literally thousands of different styles of trim today, the ones used most often are still quite simple (some common profiles are shown in the drawing at right).

STEP 6 RAISE THE WALLS

Posted by on 16/ 11/ 15

Подпись:

Подпись: 1. To mark the cut line, position the brace diagonally across the wall section from the bottom plate to the double top plate, and then pencil a line along one edge of every framing member the brace crosses. [Photo © Larry Haun] STEP 6 RAISE THE WALLS

As with barn raisings of yore, it takes a few warm bodies to raise framed walls. Let one per­son be the team leader and encourage everyone

Подпись: The first wall goes up! Make sure you have enough help when you're ready to raise the walls. Have one person take charge, and get everyone to lift in unison. [Photo by HFHI/Will Crocker] When you re­move the tem­porary blocks nailed to the rim joist, be sure to pull the nails or bend them over so that no one gets a nail in the foot!

Подпись: Temporary braces are important. After raising a wall, make sure it is braced securely with 2x lumber that extends diagonally from the rim joist or the floor to the top of a stud in the wall. Secure bracing eliminates the serious injuries that can result from a falling wall. These braces also help keep walls plumb, straight, and ready for roof trusses.Подпись: Nailing the bottom plate is best done when one worker nails while others steady the wall. The short 2x4s nailed to the rim joist prevent the bottom plate from sliding off the floor when the wall is raised.

to work together (see the photo above). Remind people to lift with their legs, not with their backs. In many areas, builders put a heavy bead of caulk or a roll of foam (polystyrene) on the floor or slab under the bottom plate before rais­ing a wall. This helps keep out cold air as well as any bugs that may want to migrate inside. To ensure that the wall won’t slip over the outside edge of the building as it’s being raised, nail pieces of 2x stock to the rim joist so they stick up a few inches above the floor to catch and hold the bottom plate (see the photo at right). On a slab, bolts hold the bottom plate in place.

Nail on the double top plate

Posted by on 16/ 11/ 15

In some parts of the country, carpenters raise the walls before cutting and nailing on the double top plate. In my opinion, the time to nail on the double top plate is now—while the wall is still flat on the floor. Otherwise, you will have to use a ladder. The top plate is an impor­tant structural member. It ties the entire frame together. Without it, severe lateral stress from an

earthquake or high winds could easily rip apart a building. If you frame with a single top plate, secure them together with metal plate straps.

On a 2×4 butt wall, the double top plate ex­tends beyond the top plate a bit less than ЗУ2 in. (cut it /4 in. short—about 3/4 in.—to make sure it doesn’t protrude beyond the through wall), so that it can tie into the notch in the double top plate of a through wall. On through walls, the double top plate has notches where it receives the double top plates extending from butt or intersecting walls. Now you can see why it was important to mark the plates accurately while locating the corners and channels. You don’t need to measure the length of double top plates. All you need to do is set the double top plate on a through wall above the top plate, with one end held back З’А in. (5У2 in. on 2×6 walls) from the end, then cut it to length.

At channel marks, leave a 33/4-in. cutout so that an intersecting wall can comfortably lap over and tie in at that spot (see the photo on p. 100). When cutting and installing double top plates, leave about a 3/4-in. gap where the walls
tie into each other. This makes it easier for the overlapping double top plates to slip into place. This is another one of those times when it’s okay to be less than absolutely accurate.

When you nail the double top plate to the top plate, it’s important to make sure that there are no gaps in the top plate. The top plate stock must butt tightly together, just as it did when you plated the walls on the subfloor. Nail the double top plate to the top plate with two 16d nails at each end of the wall and at each break in either the top plate or the double top plate. Elsewhere, nail one 16d nail over each stud.

It is best not to nail between studs, because electricians and plumbers run wires and pipes through holes drilled in those locations. Hitting a nail while drilling can dull the bit and give your arm a nasty twist.

Brace the walls

Подпись:Подпись: With the bottom plate nailed off, cut the double top plate in place, following the layout marks on the top plate.

Подпись: Alignment is important. As volunteers learn to nail studs, they often find it difficult to keep them aligned on their layout marks and to keep their edges flush with the edges of wall plates. For more accurate results, work in teams of two. Have one volunteer hold the stud on its layout while another drives the nails. Use a cat's paw to pry studs and nails loose when the alignment must be corrected.

The sudden, intense pressure exerted on a wall by a hurricane, tornado, or earthquake can be devastating. To withstand these forces, the walls must be well braced. As a novice carpenter, I

Подпись:Подпись: Install the double top plate. Gaps in the double top plate allow those in a butt wall to overlap. used to cut 2x stock with a handsaw and nail them between the studs, running it diagonally from plate to plate. It was a good brace, but it took about an hour to make each one. These days, braces are much easier to install. You can attach L-shaped metal angle braces or nail plywood or OSB sheathing to the frame. Code requirements vary, so be sure to check with your building department to find out how the walls should be braced. In some areas, bracing is also required on certain interior walls.

USING METAL ANGLE BRACES. Metal angle braces are easy to install (see the photos on the facing page). In many areas, they can be used instead of plywood or OSB sheathing.

If the finished siding you plan to install can be nailed directly to the studs or furring strips (clapboards and fiber-cement boards are good examples), metal braces can save you the
expense of plywood or OSB sheathing. Every exterior wall should receive a metal brace at every corner as well as every 25 ft. between corners. The typical metal brace has an L – shaped profile; it’s designed to be set into a kerf cut diagonally across the wall. Here’s how to install one:

1. Position the brace and mark the cut. Lay

the brace across a framed wall so that it extends at a 45-degree angle from the bottom plate to the double top plate. Trace a pencil mark along one side, as shown in the far left photo on the facing page.

2. Cut the kerf. With a circular saw, cut a l-in.-deep slot along the line into the plates and studs.

3. Nail off the bottom of the brace. Slip one

flange of the brace into the slot and nail it to the bottom plate with three 8d nails. Drive one more 8d nail through the brace and into the first stud. At the double top plate, start an 8d nail alongside the brace and bend the nail over to hold the brace in place as the wall is raised.

Now you are ready to raise and plumb the wall. It’s important not to install the brace com­pletely until after the wall has been raised and plumbed. See pp. 101—108 for details on raising and plumbing walls.

Finish nailing the brace to the studs and plates by driving one 8d nail through the brace and into every stud it crosses. At the top of the wall, drive three nails through the brace and into the top and double top plates. If the top of the brace extends above the double top plate, trim it flush with a hacksaw.

USING PLYWOOD AND OSB BRACING. When nailed properly to wall framing, plywood and OSB provide much stronger racking resis­tance than metal braces do. Wall sheathing is essential as a substrate for some types of exterior siding. It also acts as a wind and weather bar­rier. Many codes require that a full sheet of OSB be nailed at each exterior corner and every 25 ft.

along the wall. The spaces between can be filled with sheets of rigid foam insulation. That’s the sheathing strategy we used on this house.

There are different ways to install wood sheathing panels. Sometimes sheathing is positioned to extend over the wall and cover the rim joist. I try to keep OSB V2 in. away from concrete so it won’t absorb water. On a one-story building, my preference is to sheathe the walls once they are raised. This is especially true when working on a slab that has plumbing pipes sticking up.

Other builders prefer to sheathe the walls while they are flat on the floor. One of the prob­lems with sheathing the walls before raising them is that they become heavy. To raise a long 2×6 wall fully sheathed with OSB or plywood, you may have to call in the National Guard.

Or you could use a wall jack, a device that hooks under a wall and slowly raises it up (see

Resources on p. 279). Sheathing a wall while it’s still flat on the subfloor also requires greater accuracy—the wall must be dead-on straight and square before it’s sheathed. You can do this by making sure the bottom plate is directly on the chalkline and the end corner studs are flush with the outside of the building. It doesn’t hurt to measure from corner to corner to check the wall for square. After the wall is ready, you can attach the required sheets of plywood or OSB (8d nails every 6 in. o. c. around the perimeter,

12 in. o. c. in the field, or middle of the sheet). Be sure to insulate headers, corners, and channels before covering them with sheathing.

All You Need to Know about Spray Foam

Posted by on 16/ 11/ 15

■ BY ROB YAGID

I

recently spent a day pulling wire with a friend who’s an electrician in New York. Late in the afternoon, our conversation turned to a client and friend of his who was seeking advice about insulating her new home. The topic caught the interest of some other guys on site, most from differ­ent trades, who gathered around and offered their opinions on which material she should use. After a brief debate, everyone seemed confident that spray foam would yield the best performance. That was until I threw out the question, "Which type?" Sure, they all knew there were two types of spray polyure­thane foam, open cell and closed cell, but no one knew enough about them to step up and defend the use of one over the other. The truth is, neither did I.

Spray polyurethane-foam manufacturers have a relatively easy job when it comes to marketing their products because of one key statistic. According to the U. S. Department of Energy, 30% or more of a home’s heating and cooling costs are attributed to air leak­age. Spray polyurethane foam, or spray foam as it’s most often called, is an effective air

barrier and significantly reduces energy loss. Combined with a higher R-value than most other forms of insulation, it’s no wonder spray foam is often relied on to help make houses ultraefficient. Choosing to insulate your home with spray foam doesn’t guar­antee that it’ll perform to its full potential. Different climates, construction practices, and wall and roof assemblies benefit from different types of foam. The installation of foam at specific thicknesses is critical when you’re trying to get the most performance for the money.

It Won’t Settle, and It Doesn’t Off­Gas Toxic Chemicals

Because of the urea-formaldehyde foam used to insulate homes in the 1970s, which could degrade and off-gas unsafe formaldehyde, spray foam is often perceived as being un­healthful and poorly performing. Installers that look as if they’re outfitted to survive a

Подпись:

Подпись: CLOSED CELL Density: 2 lb. per cu. ft. R-value: 6 per in. (aged) Vapor permeability: Semi-impermeable
All You Need to Know about Spray Foam

nuclear catastrophe perpetuate the miscon­ception that spray foam is toxic.

The fact is that when it’s installed prop­erly, spray foam is more physically stable than the studs and sheathing it’s adhered to. The oxygen-supplied respirators and head – to-toe protective suits installers wear are necessary only to keep the chemicals that make up spray foam out of their lungs and off their skin during installation.

The blowing agent, a gas that expands the foam’s cells to give it volume, receives a lot of scrutiny. Over time, from three months to a year, a portion of the blowing agent in closed-cell foam evaporates into the air. Prior to 2003, chlorofluorocarbon and hydrochlorofluorocarbon blowing agents were in widespread use. These gases are dam­aging to the atmosphere. The U. S. Environ­mental Protection Agency has banned the use of those chemicals and recognized the current hydrofluorocarbon (HFC) blowing agent as a safe alternative.

Open-cell foam, which uses water as its blowing agent, emits carbon dioxide as it expands. But manufacturers claim that the amount of carbon dioxide released from the foam has a limited impact on the environ­ment. The Spray Polyurethane Foam Alli­ance is currently testing this issue.

BUILDING CORNERS AND CHANNELS

Posted by on 16/ 11/ 15

Like headers, outside corners and channels can be built in a number of different ways, and preferences tend to be regional. The most common constructions are shown in the illustration below. A two-stud outside corner works well in most cases, but if you are planning to install clapboard or fiber-cement siding directly to the studs (with no exterior sheathing), you’ll need more backing at the outside corners. A blocked-up corner provides more backing and mak es good use of 2x offcuts. In addition, all of these outside cor­ner configurations provide backing on the inside of the wall for drywall or other wallboard.

Channels (sometimes called tees) are most easily made with a flat stud or blocks nailed between two regular studs. I place a crowned or knot-filled stud between two good studs. In some parts of the country, framers build ladder-type chan­nels to provide backing at intersecting walls. Building ladder backing doesn’t save a lot on materials, but it does allow more insulation to be inserted at those spots.

Both corners and channels are nailed together with one 16d nail every 2 ft. o. c. When nailing channels to the top and bottom plates, make sure that the flat stud is oriented cor­rectly to provide backing for an intersecting wall. It’s not dif­ficult to put in a channel upside-down.

BUILDING CORNERS AND CHANNELS

This corner saves a 2x stud and allows for more insulation in the wall.

 

An outside corner can be made with two studs and three short blocks.

 

A channel can be made from three studs or from two studs and three short, flat blocks nailed between them.

 

A channel made with short 2xs nailed between the studs allows the area to be well insulated.

 

BUILDING CORNERS AND CHANNELS

BUILDING CORNERS AND CHANNELS

explains the main types of corners and channels. You can build corners and channels in place as you frame the walls, or you can build them all at once in a central location, then distribute them and insert them in the walls as needed.

Keep your eyes open for studs that are bowed, twisted, or crowned. Set them aside to use for blocking and roof braces. This ensures a more uniform finished wall and makes it easier for
finish carpenters to install cabinets, countertops, and interior trim. It’s always good to think about how to make future tasks easier. So take care to use straight studs in all walls that will hold kitchen cabinets.

Nail on the top and bottom plates

It’s finally time to pry apart the two wall plates that were tacked together on the subfloor.

Подпись: A pneumatic nailer makes quick work of making a channel, which consists of two studs separated by 2x4 blocking. Подпись:BUILDING CORNERS AND CHANNELSПодпись: If possible, nail the blocks for cabinets, toilet fixtures, and even the closets to the wall before it has been positioned upright. It's much easier to install the blocks when the wall is flat on the deck.

Working on one wall at a time, separate the top wall plate from the bottom plate, removing the 8d tack nails as you go. Leave the bottom plate tacked to the floor for now. Nail the top plate first, using the bottom plate to keep the studs aligned. Move the top plate straight up to the upper ends of the studs. Don’t turn a top plate end for end as you move it into position or you’ll have a real mess. The exterior walls can be framed over the interior walls rather than flat on the deck or slab (see the top photo above). This is okay. The most important ele­ment in wall framing is to keep the frame reasonably straight and square on the floor.

This is not difficult to do. Take your time until you get it right.

Begin nailing studs to the plate at an outside corner. Make each stud flush with the edge of the plate. Drive a pair of 16d nails through the plate into each stud. You will find that consis­tently working either from left to right or from right to left has a natural feel. Over time, you will develop a rhythm to nailing studs that involves not just your hands and arms but your entire body.

If you are using a pneumatic nailer, always drive the bottom nail first. Then remove your hand from the stud and drive the top nail. If you keep your hand in place while driving the top nail, sooner or later you will miss and drive a nail into your hand. Unfortunately, I learned this the hard way.

When you come to a door or window open­ing, be especially careful to nail each king stud on the “X” mark next to the header location. Once all the wall, king, and top cripple studs are nailed to the top plate, pull up the bottom plate and begin nailing it in place, always pay­ing attention to your layout marks. Repeat the process. Nail all wall members to the bottom plate just like you did with the top plate.

I always nail the blocks for a recessed, or in-wall, medicine cabinet while the wall is flat on the floor. At the “MC” marking, hook your tape on the bottom plate and measure up 4 ft. and 6 ft. on the two studs on each side. Nail a flat block below the 4-ft. marks and above the 6-ft. marks. This leaves a clear space of 2 ft., which is the height of a standard recessed medicine cabinet.

Analytical Approaches

Posted by on 16/ 11/ 15
Analytical Approaches Подпись: в Подпись: (3.5)

An alternative to the graphic technique is to estimate the statistical parameters of a distribution from the sample data (refer to Sec. 3.6). Then the distribution model can be used to solve for the variate value corresponding to any desired return period or probability as

in which F-1(e) is the inverse cumulative distribution function with the model parameter vector в. Equation (3.5) can be applied when the inverse distribu­tion function forms are analytically amenable, such as for the Gumbel, gener­alized extreme value, generalized logistic, and generalized Pareto distributions (see Sec. 2.6.6).

Example 3.2 Consider that the annual maximum floods follow a lognormal distribu­tion with a mean of 490 ft3/s and a standard deviation of 80 ft3/s. Determine the flood magnitude with a 1-in-100 chance of being exceeded in any given year.

Solution From Eqs. (2.67a) and (2.67b), the parameters of a lognormal distribution, for annual maximum flood Q, can be obtained as

Подпись: = 0.1622Obq = ^ln (vQ +[5]) = ^ln (490) + 1 Mnq = ln(MQ) – 1 Oj2n Q = ln(490) – 1(0.1622)2 = 6.1812

Since ln( Q) follows a normal distribution with a mean of mn q = 6.1812 and a stan­dard deviation of Oln q = 0.1622 as previously computed, the magnitude of the log – transformed 100-year flood can be calculated by

ln(g100)—= Ф-1 f 1 – -^ = Ф-1(0.99) = 2.34

Oln Q V 100 у

Hence ln(^100) = Mln Q + 2.34 x Olnq = 6.5607, and the corresponding 100-year flood magnitude can be calculated as дю0 = exp[ln(q10Q)] = 706.8 ft3/s.

For some distributions, such as Pearson type 3 or log-Pearson type 3, the appropriate probability paper or CDF inverse form is unavailable. In such a case, an analytical approach using the frequency factor KT is applied:

XT = l^x + Kt x ax (3.6)

in which xT is the variate corresponding to a return period of T, /гх and oX are the mean and standard deviation of the random variable, respectively, and KT is the frequency factor, which is a function of the return period T or P (X > xT) and higher moments, if required. It is clear that a plot of Eq. (3.6) (xT versus KT)
on linear graph paper will yield a straight line with slope of ax and intercept l^x at Kt = 0.

In order for Eq. (3.6) to be useful, the functional relationship between Kt and exceedance probability or return period must be determined for the distribution to be used. In fact, the frequency factor Kt = (xT — xx)/ax is identical to a standardized variate corresponding to the exceedance probability of 1/T for a particular distribution model under consideration. For example, if the normal distribution is considered, then Kt = zT = Ф—41 — T —:). The same applies to the lognormal distribution when the mean and standard deviation of log – transformed random variables are used. Hence the standard normal probability table (Table 2.2) provides values of the frequency factor for sample data from normal and log normal distributions. Once this relation is known, a nonlinear probability or return-period scale can be constructed to replace the linear Kt scale, and thus special graph paper can be constructed for any distribution so that plot of xT versus P or T will be linear.

Gumbel probability paper has been printed, although it is not readily avail­able from commercial sources. Referring to Eq. (2.85a), the relationship between Kt and T for this distribution can be derived as

Analytical Approaches

V6

n

 

T

T – 1

 

Kt

 

0.5772 + ln

 

(3.7)

 

Analytical Approaches

Подпись: KT (YX) = — Yx Подпись: 1 + ZT Подпись: Yx ~6 Подпись: Yx ~6 Подпись: 21 3 1 Подпись: (3.8)

For Pearson and log-Pearson type 3 distributions, linearization can be ac­complished according to Eq. (3.6). However, for this distribution, the frequency factor is a function of both P or T and the skewness coefficient yx. This means that a different nonlinear P or T scale is required for each skewness coeffi­cient, and therefore, it is impractical to construct a probability paper for this distribution. However, it should be pointed out that if yx = 0 in log-space, the log-Pearson type 3 reduces to the lognormal distribution, and thus commercial lognormal probability paper can be used. The relationship between frequency factor Kt, T, and yx cannot be developed in a closed form, as was done for the Gumbel distribution in Eq. (3.7). However, the relationship can be computed numerically, and the results are given in Table 3.3. For 0.99-1 < T < 100 and |yx | < 2, the frequency-factor values are well approximated by the Wilson – Hilferty transformation (Stedinger et al., 1993):

in which zT is the standard normal quantile with exceedance probability of 1/T.

The procedure for using the frequency-factor method is outlined as follows: 1

Return period in years

Skewness coefficient yx

2

5

10 25 50 Exceedence probability

100

200

0.50

0.20

0.10

0.04

0.02

0.01

0.005

3.0

-0.396

0.420

1.180

2.278

3.152

4.051

4.970

2.9

-0.390

0.440

1.195

2.277

3.134

4.013

4.909

2.8

-0.384

0.460

1.210

2.275

3.114

3.973

4.847

2.7

-0.376

0.479

1.224

2.272

3.093

3.932

4.783

2.6

-0.368

0.499

1.238

2.267

3.071

3.889

4.718

2.5

-0.360

0.518

1.250

2.262

3.048

3.845

4.652

2.4

-0.351

0.537

1.262

2.256

3.023

3.800

4.584

2.3

-0.341

0.555

1.274

2.248

2.997

3.753

4.515

2.2

-0.330

0.574

1.284

2.240

2.970

3.705

4.444

2.1

-0.319

0.592

1.294

2.230

2.942

3.656

4.372

2.0

-0.307

0.609

1.302

2.219

2.912

3.605

4.298

1.9

-0.294

0.627

1.310

2.207

2.881

3.553

4.223

1.8

-0.282

0.643

1.318

2.193

2.848

3.499

4.147

1.7

-0.268

0.660

1.324

2.179

2.815

3.444

4.069

1.6

-0.254

0.675

1.329

2.163

2.780

3.388

3.990

1.5

-0.240

0.690

1.333

2.146

2.743

3.330

3.910

1.4

-0.225

0.705

1.337

2.128

2.706

3.271

3.828

1.3

-0.210

0.719

1.339

2.108

2.666

3.211

3.745

1.2

-0.195

0.732

1.340

2.087

2.626

3.149

3.661

1.1

-0.180

0.745

1.341

2.066

2.585

3.087

3.575

1.0

-0.164

0.758

1.340

2.043

2.542

3.022

3.489

0.9

-0.148

0.769

1.339

2.018

2.498

2.957

3.401

0.8

-0.132

0.780

1.336

1.993

2.453

2.891

3.312

0.7

-0.116

0.790

1.333

1.967

2.407

2.824

3.223

0.6

-0.099

0.800

1.328

1.939

2.359

2.755

3.132

0.5

-0.083

0.808

1.323

1.910

2.311

2.686

3.041

0.4

-0.066

0.816

1.317

1.880

2.261

2.615

2.949

0.3

-0.050

0.824

1.309

1.849

2.211

2.544

2.856

0.2

-0.033

0.830

1.301

1.818

2.159

2.472

2.763

0.1

-0.017

0.836

1.292

1.785

2.107

2.400

2.670

0.0

0

0.842

1.282

1.751

2.054

2.326

2.576

-0.1

0.017

0.846

1.270

1.716

2.000

2.252

2.482

-0.2

0.033

0.850

1.258

1.680

1.945

2.178

2.388

-0.3

0.050

0.853

1.245

1.643

1.890

2.104

2.294

-0.4

0.066

0.855

1.231

1.606

1.834

2.029

2.201

-0.5

0.083

0.856

1.216

1.567

1.777

1.955

2.108

-0.6

0.099

0.857

1.200

1.528

1.720

1.880

2.016

-0.7

0.116

0.857

1.183

1.488

1.663

1.806

1.926

-0.8

0.132

0.856

1.166

1.448

1.606

1.733

1.837

-0.9

0.148

0.854

1.147

1.407

1.549

1.660

1.749

-1.0

0.164

0.852

1.128

1.366

1.492

1.588

1.664

-1.1

0.180

0.848

1.107

1.324

1.435

1.518

1.581

-1.2

0.195

0.844

1.086

1.282

1.379

1.449

1.501

-1.3

0.210

0.838

1.064

1.240

1.324

1.383

1.424

-1.4

0.225

0.832

1.041

1.198

1.270

1.318

1.351

-1.5

0.240

0.825

1.018

1.157

1.217

1.256

1.282

-1.6

0.254

0.817

0.994

1.116

1.166

1.197

1.216

Return period in years

Skewness coefficient yx

2

5

10 25 50 Exceedence probability

100

200

0.50

0.20

0.10

0.04

0.02

0.01

0.005

-1.7

0.268

0.808

0.970

1.075

1.116

1.140

1.155

-1.8

0.282

0.799

0.945

1.035

1.069

1.087

1.097

-1.9

0.294

0.788

0.920

0.996

1.023

1.037

1.044

-2.0

0.307

0.777

0.895

0.959

0.980

0.990

0.995

-2.1

0.319

0.765

0.869

0.923

0.939

0.946

0.949

-2.2

0.330

0.752

0.844

0.888

0.900

0.905

0.907

-2.3

0.341

0.739

0.819

0.855

0.864

0.867

0.869

-2.4

0.351

0.725

0.795

0.823

0.830

0.832

0.833

-2.5

0.360

0.711

0.771

0.793

0.798

0.799

0.800

-2.6

0.368

0.696

0.747

0.764

0.768

0.769

0.769

-2.7

0.376

0.681

0.724

0.738

0.740

0.740

0.741

-2.8

0.384

0.666

0.702

0.712

0.714

0.714

0.714

-2.9

0.390

0.651

0.681

0.683

0.689

0.690

0.690

-3.0

0.396

0.636

0.666

0.666

0.666

0.667

0.667

SOURCE: U. S. Water Resources Council (1981).

2. For the desired return period, determine the associated value of KT for the distribution.

3. Compute the desired quantile value using Eq. (3.6) with x replacing цx and sx replacing ax, that is,

XT = x + KT x sx (3.9)

It should be recognized that the basic difference between the graphic and analytical approaches is that each represents a different method of estimating the statistical parameters of the distribution being used. By the analytical ap­proach, a best-fit line is constructed that then sets the statistical parameters. In the mathematical approach, the statistical parameters are first computed from the sample, and effectively, the line thus determined is used. The line deter­mined using the mathematical approach is in general a poorer fit to the observed data than that obtained using the graphic approach, especially if curve-fitting procedures are applied. However, the U. S. Water Resources Council (1967) rec­ommended use of the mathematical approach because

1. Graphic least-squares methods are avoided to reduce the incorporation of the random characteristics of the particular data set (especially in the light of the difficulty in selecting the proper plotting-position formula).

2. The generally larger variance of the mathematical approach is believed to help compensate for the typically small data sets.

Example 3.3 Using the frequency-factor method, estimate the flows with return pe­riods of 2, 10, 25, 50, and 100 years for the Boneyard Creek using the Gumbel and log-Pearson type 3 distributions. Use the historical data in Example 3.1 as a basis for the calculations.

Solution Based on the samples, the method requires determination of the frequency factor Kt in

xt = x + Kt x sx

Analytical Approaches
Подпись: (6361.8)1/2 = 79.8ft3/s

For the Gumbel distribution, values of Kt can be calculated by Eq. (3.7). For the log-Pearson type 3 distribution, Table 3.3 or Eq. (3.8) can be used, which requires computation of the skewness coefficient. The calculations of relevant sample moments are shown in the following table:

Year

Original scale

Log – Transformed scale

gi (ft3/s)

gi2

gi3

Уі = ln( gi)

Уі2

Уі3

1961

390

1.52e + 05

5.93e + 07

5.97

35.59

212.36

1962

374

1.40e + 05

5.23e + 07

5.92

35.10

207.92

1963

342

1.17e + 05

4.00e + 07

5.83

34.05

198.65

1964

507

2.57e + 05

1.30e + 08

6.23

38.79

241.63

1965

596

3.55e + 05

2.12e + 08

6.39

40.84

260.95

1966

416

1.73e + 05

7.20e + 07

6.03

36.37

219.33

1967

533

2.84e + 05

1.51e + 08

6.28

39.42

247.50

1968

505

2.55e + 05

1.29e + 08

6.22

38.75

241.17

1969

549

3.01e + 05

1.65e + 08

6.31

39.79

251.01

1970

414

1.71e + 05

7.10e + 07

6.03

36.31

218.81

1971

524

2.75e + 05

1.44e + 08

6.26

39.21

245.49

1972

505

2.55e + 05

1.29e + 08

6.22

38.75

241.17

1973

447

2.00e + 05

8.93e + 07

6.10

37.24

227.27

1974

543

2.95e + 05

1.60e + 08

6.30

39.65

249.70

1975

591

3.49e + 05

2.06e + 08

6.38

40.73

259.92

Sum =

7236

3.58e + 06

1.81e + 09

92.48

570.58

3522.88

For the Gumbel distribution,

For the log-Pearson type 3 distribution,

Eln(gf) 92.48

 

6.165

 

У =

n

15

sy =

1—1 1

1 1

gy =

n

m3

(n – 1)(n – 2) syj

 

= (0.417/14)1/2 = 0.173

 

15(-0.00336) (14)(13)(0.173)3

 

-0.540

 

in which m3 = Уз — Уі + 2ny3. The determination of the values of frequency

factor corresponding to different return periods is shown in the following table:

Frequency factor by distribution

Return period

Exceedance

Nonexceedance

Gumbel

LP3

(years)

probability

probability

Eq. (3.7)

Eq. (3.8)

2

0.50

0.50

—0.1643

0.0892

10

0.10

0.90

1.3046

1.2093

25

0.04

0.96

2.0438

1.5526

50

0.02

0.98

2.5923

1.7570

100

0.01

0.99

3.1367

1.9292

Based on the preceding frequency-factor values, the flood magnitude of the various

return periods

can be determined as

Frequency curves

by distribution (ft3/s)

Return period

Gumbel

LP3

(years)

qT = 482.4 + 79.8Kt, EV1

qT = exp(6.165 + 0.173Kt, LP3)

2

469.3

483.3

10

586.5

586.4

25

645.4

622.2

50

689.2

644.5

100

732.6

663.9

One could compare these results for the Gumbel distribution with those obtained from the graphic approach of Example 3.1.

Fine Aggregate and Filler

Posted by on 16/ 11/ 15

According to concept of van de Ven et al. (2003), an SMA mixture probably has no real stone skeleton immediately after compaction. A real skeleton in SMA is cre­ated during service under the effects of traffic and climatic loading when sand and filler grains between the coarse aggregates (skeleton) may be crushed or moved. Accordingly, at the design stage, the content of fine aggregate and filler must be determined.

Cause-and-effect relationships between the filler and the fine aggregate (crushed sand) have not been determined in a design method. Some Dutch research into this has led to establishing the optimal relationship between those elements. Research on sand-filler mixes and the filling and replacing effects occurring between them have been elaborated on in a Dutch publication (Voskuilen, 2000). This effect is shown in Figure 7.13; its description is as follows:

• The compacted fine aggregate (crushed sand) contains a quantity of air voids.

• As filler (particles smaller than 0.063 mm) is gradually added, it fills the air voids in the sand, and the voids in the sand-filler mix get smaller. This is the filling-stage; the existing skeleton of the mix is made of sand (the shaded area in Figure 7.13);

• The decrease of air voids continues until the voids in the sand are com­pletely filled (reaching the minimum possible); then only air voids in the filler remain;

• The further addition of filler with a simultaneous decrease in the amount of sand causes a gradual increase of air voids in the mix. This is the replace­ment stage; the existing skeleton of the mix is made of filler (the clear area in Figure 7.13), and grains of sand are being shoved aside by filler particles.

We have found the root of the aformentioned effect—after all, it accompanies the supplementing of fine aggregate to coarse grains in SMA—from the gradual
decrease of air voids, through the void’s minimum, up to the gradual skeleton open­ing (as described in Chapter 6 in Section 6.2.3 on binary systems).

Looking at the form of the example in Figure 7.13, which shows the connection between the filler quantity and the content of air voids, we can observe that the rate of decrease of air voids is faster in the filling phase than its increase in the replacement phase. Thus, in the case of necessary adjustments to the content of air voids, a change in the sand fraction content will produce a stronger effect than will altering the filler content (leaving the chipping fraction unchanged) (Voskuilen, 2000).

According to Dutch research (Voskuilen, 2000), the recommended ratio of the quantity of fine aggregate (sand) to the quantity of filler amounts is 65:35 (m/m). If the filler density is about 2.700 g/cm3 and the density of crushed sand is about 2.650 g/cm3, this proportion may be employed without recalculation. Mass proportions should be converted into volume proportions in cases of significant deviations from these density values.

We were aware of air voids among coarse aggregate some time ago; now that we have set the sand-filler ratio, we can determine the total mastic volume in the SMA. The mastic volume is calculated according to the formula (Voskuilen, 2000)

Подпись: Vmimage75
pb pf ps pa

mb = Binder mass, % (m/m)

pb = Binder density, g/cm3

mf = Filler mass, % (m/m)

pf = Filler density, g/cm3

ms = Sand fraction mass, % (m/m)

ps = Sand fraction density, g/cm3

ma = Stabilizer (drainage inhibitor) mass, % (m/m)

pa = Stabilizer (drainage inhibitor) density, g/cm3

The filling ratio stone skeleton (FRs) is used in the Dutch method to determine the theoretical degree of filling of the air voids in the coarse-aggregate skeleton with mastic (i. e., for investigating whether the design mastic volume is an optimal one). FRs is defined with the formula

V – V

FRs = -^—^ -100%
Vs

FRs = Percentage ratio of filling the coarse-aggregate skeleton with mastic, % (v/v)

Vm = Mastic volume, % (v/v)

Vs = Air voids in the compacted coarse-aggregate skeleton, % (v/v)

Air voids in the compacted coarse-aggregate skeleton (Vs) are calculated using the formula

Vs = pg pg -100% pg

pg = Density of the coarse aggregate fraction, g/cm3

pb = Bulk density of the coarse aggregate fraction compacted in a gyratory com­pactor with a lubricating agent, g/cm3

The assessment of FRs ratio is as follows:

FRs < 0 implies that the air voids are not filled with enough mastic.

FRs = 0 implies that the air voids are filled with the mastic.

FRs > 0 implies that the air voids are overfilled with mastic.

For every SMA design, the FRs ratio should not exceed 0. One should remember that this is a theoretical factor and does not take into consideration the enlarging effect of the increasing air voids in the coarse-aggregate skeleton. Due to this, compacted SMA mixtures with air void contents of 4-5% (v/v) can all be marked by FRs = -4 (Jacobs and Voskuilen, 2004). It is easy to see that the content of the coarse-aggregate fraction and the size of air voids in the coarse-aggregate skeleton are dependent on the FRs level.

Drainage and land improvement in the Mycenaen civilization

Posted by on 16/ 11/ 15

Many of the regions of Peloponnese or Attica are karsitic. Entire rivers disappear into abysses or caverns (called catavothres in Greek), only to reappear at some distant point.

When the subterranean cavities fill up or are blocked, for example after earthquakes, water can accumulate in marshes and lakes, the water level varying from season to sea­son and from one period to the next. Strabo describes these phenomena:

“Some of these plains are marshy, since rivers spread out over them, though other rivers fall into them and later find a way out; other plains are dried up, and on account of their fertility are tilled in all kinds of ways. But since the depths of the earth are full of caverns and holes, it has often happened that violent earthquakes have blocked up some of the passages, and also opened up others, some up to the surface of the earth and others through underground chan­nels. The result for the waters, therefore, is that some of the streams flow through under­ground channels, whereas others flow on the surface of the earth, thus forming lakes and rivers. And when the channels in the depths of the earth are stopped up, it comes to pass that the lakes expand as far as the inhabited places, so that they swallow up both cities and dis­tricts, and that when the same channels, or others, are opened up, these cities and districts are uncovered; and that the same regions at one time are traversed in boats and at another on foot, and the same cities at one time are situated on the lake and at another far away from it.”[151]

To be arable, these valleys had to be drained and the lake levels stabilized. The most important of such efforts were developed in Beotia by the Mynians (subjects of the king Mynias), near Orchomenos, their capital. The memory of Mynian power and manage­ment of the lake Copais was still fresh at the time of Strabo:

“They say that the place now occupied by lake Copais used to be dry, that it then belonged to the Orochomenians, their close neighbors, and that all sorts of crops were grown there. Here one sees an additional confirmation of the wealth of this city.”[152]

Lake Copais is fed by runoff from rainfall and by the Kephissos river. The natural grottos already mentioned, i. e. catavothres, were used to drain it to the sea.[153] The waters of the Kephissos, previously flowing into the lake, were detoured to an under­ground passage through a 25-km long canal. The canal may have also been used for nav­igation. The land reclaimed by emptying of the lake was itself drained, surrounded by protective dikes, and brought under cultivation. Water from what remained of the lake provided irrigation for these lands. The Mynians built the palace of Gla (Homer’s Arne) in one area reclaimed from the lake, in the middle of the depression. The lake, now fed only by runoff from rainfall, was nearly dry in the summer, so that part of its bed could also be cultivated. Figure 4.10 is a map of developments around the lake that were very likely in operation about 1300 BC. With the end of the Mycenaen civilization, the lake’s systems were no longer maintained, and the marshy lake reestablished itself. Between 334 and 331 BC one of Alexander the Great’s engineers, a certain Crates of Chalchis, again set out to drain and dry the lake. But these efforts were not completed, either because of technical difficulties in the region or some other troubles.

Drainage and land improvement in the Mycenaen civilization

1994).

These same techniques are used to reclaim marshy valleys in many other locations. Typically there are dikes surrounding a reservoir-lake fed by rainwater and snowmelt, the dikes also serving to protect dry areas that are drained into grottos or caverns. Eight such reservoirs, including the Boedria reservoir shown in Figure 4.10, are listed in table 4.1. Five of these sites are in Peloponnese (Figure 4.6). For all of these projects, the dikes are between two and four meters high, and of variable length from 200 to 2,500 m. They most often are built of earth fill between two exterior walls. Some of these dikes are still visible today (Figure 4.11).

Table 4.1 Mycenaen dam-reservoirs (after Knauss, 1991; Schnitter, 1994)

Name

(see Figure 4.6)

Height

(m)

Length

(m)

Reservoir Volume

(million m3)

Boedria

2

1,250

24

Kineta (Thisbe)

2.5

1,200

4

Mantinea

3

300

15

Orchomenos

2

2,100

16

Permessos

4

200

2

Pheneos

2.5

2,500

19

Stymphalos

2.5

1,900

9

Takka

2

900

9

Drainage and land improvement in the Mycenaen civilization

Figure 4.11 Remains of the Kineta dam (Thisbe), 1,200 m long. The modern road was built on top of the ancient dam; at the left, remains of the wall of large cut stone (photo by the author).

Port development at Pylos

Posted by on 16/ 11/ 15

Between 1400 and 1200 BC, Achean shipping dominates long-distance commerce in the eastern Mediterranean, extending to Sicily, and perhaps even as far as to Spain. The ports of Antiquity are often developed in natural bays (that do not always provide good shelter), or in river mouths.

Recently, a detailed study of the Pylos region has made it possible to reconstitute the development of an artificial port.[150] This port was created by excavating a closed basin into marine sediments, and linking it to the sea through a channel (Figure 4.8, 4.9). The sinuous path of the channel keeps ocean swells from entering the port itself.

The port would rapidly have become unusable without additional engineering efforts, either due to silting-in of the basin itself, or by the blocking of the entrance chan-

Подпись: Figure 4.8. The artificial port of Pylos (1400 to 1200 BC) (after Shelmerdine, 1997).

nel by wave-transported sand. A through-flow was necessary to keep the port open, as would be the case in the natural estuary of a river. Therefore a river called the Selas, nat­urally flowing into the Osmanaga pond to the south, was relocated so that part of its flow, very likely controlled, passed through the port on its way to the sea. An artificial lake, linked to the port by a canal dug through a rocky barrier, served as an intermediate stor­age and settling basin for the river flow.

Подпись: Figure 4.9 The site of the ancient port of Pylos from the heights to the east of the entrance canal, looking to the north; the flat area with the orchard is the ancient basin of the port (photo by the author).
Port development at Pylos

Study of the history of sedimentation in the Osmanaga pond enables us to date this wonderful project to about 1200 BC. Such analysis also suggests that after the destruc­tion of Pylos, in about 1200 BC, the totality of the river flow takes the direct path to the sea through the port.