CORROSION PROTECTION OF NEW STEEL BRIDGES
The application of protective coatings to steel bridges, and the maintenance reapplication of coatings, is costly and so alternatives to the use of coated steel should be sought. Where appropriate, unpainted weathering steel should be used instead (see Art. 4.13). If a coated bridge is still the best candidate for the particular location, a long-lasting coating system should be applied.
Modern Paint Systems. The development of high-performance paint systems for new bridges has resulted mostly in two – or three-coat systems involving combinations of various materials including organic and inorganic zinc, epoxy, and urethane. The prime coats of these systems require cleaning the steel to a white or near white condition, which is an expensive operation even when done conveniently in a steel-fabricating plant. The application of subsequent coats, especially field coats, is labor-intensive. Despite these factors, these new systems can provide acceptably economical protection.
Water-Based Paint. The most recent emphasis in the development of paint systems has been on water-based paints. Because they do not contain volatile organic compounds, water-based paints can easily conform to the environmental restrictions placed on the levels of those compounds emitted during the painting process. Evaluation of this system and other systems continues. At this time, one can say that there is no one single paint system that is the best and most economical for all exposures. If a department of transportation or another agency representing an area with diverse geography, climate, or industrial development dictates a single-paint system for all parts of that area, it is likely that some of the bridges will be overprotected and some underprotected.
Galvanizing. Depending on local availability of galvanizing facilities of adequate size, steel members of limited length can be hot-dip galvanized. In addition to the deposition of zinc, the galvanizing process results in a change in chemistry of the surface of the steel, where an alloy is created, so that a degree of protection remains after the zinc coating is gone. The different stages of loss of coating and rusting that will eventually occur on galvanized steel can be seen on exposed highway hardware such as galvanized steel roadside barriers, luminaire supports, and traffic sign and signal supports. Since these structures are more exposed to salt spray than a bridge superstructure may be, unless the bridge is a grade-separation structure, the longevity of the protection may be expected to be greater on a bridge.
Fusion-Bonded Coating. The coating of large structural members by fusion bonding with epoxy or other powders is now feasible in at least one coating plant, but this method of coating has not been used extensively for bridges. It is frequently used for pipe piles.
Metallizing. Another method of coating steel, which has been used on small components of new bridges, such as bearing plates, and on a few existing bridges, is application of a metallic coating by the flame spray method, or “metallizing.” The existing steel is first prepared to a near white condition. Then a continuously fed wire is vaporized in a flame and sprayed onto the surface of the steel. Although results have been satisfactory, the cost on complete bridges has been extremely high compared with other methods of coating.
Selection of Protection System. Environmental conditions and owners’ experience may dictate the selection of a corrosion protection system. Where acceptable life of protection can be expected from galvanizing, painting, or use of unpainted weathering steel, the selection may be based on initial or life-cycle cost. Alternative bids should be encouraged.



















 During my researches, I have found writers (some of them engineers) who say that the over-hanging part should be one-third of the supported apart. Others say 40 percent. Some say 50 percent is the absolute limit. Let us think of these numbers as parameters. Personally, I see no reason in house-
During my researches, I have found writers (some of them engineers) who say that the over-hanging part should be one-third of the supported apart. Others say 40 percent. Some say 50 percent is the absolute limit. Let us think of these numbers as parameters. Personally, I see no reason in house-


 You could put a plank-and-beam roof on the lower frame of a building like our garage, but we chose premade engineered roof trusses to support our shingled roof. The convenience, economy, and strength characteristics of pre-built engineered trusses cannot be over-emphasized, and I will speak more of them in Chapter 4.
You could put a plank-and-beam roof on the lower frame of a building like our garage, but we chose premade engineered roof trusses to support our shingled roof. The convenience, economy, and strength characteristics of pre-built engineered trusses cannot be over-emphasized, and I will speak more of them in Chapter 4.
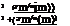
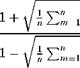 (3.18)
(3.18)