|
P |
lumbing and pipe fitting are similar, but not always the same. Modern plumbers usually work with copper tubing and various forms of plastic piping. Cast-iron pipe is still encountered, and steel pipe is used for gas work. Finding a plumber working with threaded joints is not nearly as common as it once was. But, threaded pipe is still used in plumbing, and it is used frequently in pipe fitting. Figuring the fit for a pipe where threads are to be inserted into a fitting is a little different from sliding copper or plastic pipe into a hub fitting. However, many of the calculations used with threaded pipe apply to other types of pipe.
Many plumbers don’t spend a lot of time using mathematical functions to figure offsets. Heck, I’m one of them. How often have you taken a forty-five and held it out to guesstimate a length for a piece of pipe? If you have a lot of experience, your trained eye and skill probably gave you a measurement that was close enough for plastic pipe or copper tubing. I assume this because I do it all the time. But, there are times when it helps to know how to use a precise formula to get an accurate measurement.
The need for accuracy is more important when installing threaded pipe. For example, you can’t afford to guess at a piece of gas pipe and find out the hard way that the threads did not go far enough into the receiving fitting.
In the old days, when I was first learning the trade, plumbers taught their helpers and apprentices. Those were the good-ole days. In today’s competitive market, plumbing companies don’t spend nearly as much time or money training their up-and-coming plumbers. As the owner of a plumbing company, I understand why this is, but I don’t agree with it. And, the net result is a crop
17
of plumbers who are not well prepared for what their trade requires. Sure, they can do the basics of gluing, soldering, and simple layouts, but many of the new breed don’t possess the knowledge needed to be true master plumbers. Don’t get me wrong; it’s not really the fault of the new plumbers. Responsibility for becoming an excellent plumber rests on many shoulders.
ideally, plumbing apprentices and helpers should have classroom training. Company supervisors should authorize field plumbers some additional time for in-the-field training for apprentices. Working apprentices should go the extra mile to do research and study on their own. When I was helper, I used to spend my lunch break reading the codebook. There is no single individual to blame for the quality of education that some new plumbers are, or are not, receiving. Money is probably the root of the problem. Customers are looking for low bids. Contractors must be competitive, and this eliminates the ability to have a solid on-the-job training program. Many helpers today seem to be more interested in getting their check than getting an education. So, here we are, with a lot of plumbers who don’t know the inner workings of the finer points of plumbing.
I was fortunate enough to be what might have been the last generation of plumbers to get company support in learning the trade. Plenty of time was spent running jackhammers and using shovels, but my field plumber took the time to explain procedures to me. I learned quickly how to plumb a basic house. Then I learned how to run gas pipe and to do commercial buildings. As a part of my learning process, I read voraciously. Later I became a supervisor, then the owner of my own company, and eventually an educator for other plumbers and for apprentices. I could have stopped anywhere along the way, but I’ve taken my interest in the trade to the limits, and I continue to push ahead. No, I don’t know all there is to know, but I’ve worked hard to gain the knowledge I have. Now is the time for me to share my knowledge of pipe fitting math with you.
Offsets for 45° bends are common needs in both plumbing and pipe fitting. In fact, this degree of offset is one of the most common in the trade. I mentioned
|
earlier that many plumbers eyeball such offset measurements. The method works for a lot of plumbers, but let’s take a little time to see how the math of such offsets can help you in your career.
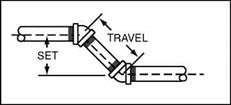
To start our tutorial, let’s discuss terms that apply to offsets. Envision a horizontal pipe that you want to install a 45° offset in. For the ease of vision, think of the horizontal pipe resting in a pipe hanger. You have to offset the pipe over a piece of ductwork. This will have a 45° fitting looking up from your horizontal pipe. There will be a piece of pipe in the upturned end of the fitting that will come into the bottom of the second 45° fitting (Fig. 2.1: offset drawing).
As we talk about measurements here, they will all be measured from the center of the pipe. There are two terms you need to know for this calculation.
Travel, the first term, is the length of the pipe between the two 45° fittings. The length of Travel begins and ends at the center of each fitting. The distance from the center of the lower horizontal pipe to the center of the upper horizontal pipe is called the Set. Now that you know the terms, we can do the math.
To make doing the math easier, I am including tables for you to work from (Fig. 2.2: 45° offset math tables). Let’s say that the Set is 563/4 inches. Find this measurement in the table in Fig. 2.2. This will show you that the Travel is 80.244 inches. Now you can use the table for converting decimal equivalents of fractions of an inch (Fig. 2.3: decimal equivalents of fractions of an inch) to convert your decimal, the 80.244 inches. Finding the decimal equivalent of a fraction is a matter of dividing the numerator by the denominator. The chart in Fig. 2.3 proves the measurement to be 80V4 inches. You can find the Set if you know the Travel by reversing the procedure.
If the Travel is known to be 80V4 inches, what is the Set? We both know that it is 563/4 inches, but how would you find it? Use the table in Fig. 2.2 and look under the heading of Travel. Find the 80.244 listing that represents 80V4 inches. Refer to the Set heading. What does it say? Of course, it says 563/4. It’s that easy. All you have to do is use the tables that I’ve provided to make your life easier in calculating 45° offsets.
|
Set |
Travel |
Set |
Travel |
Set |
Travel |
|
2 |
2.828 |
У, |
15.907 |
Vh |
28.987 |
|
‘/4 |
3.181 |
y2 |
16.261 |
3/4 |
29.340 |
|
y2 |
3.531 |
% |
16.614 |
21 |
29.694 |
|
3/4 |
3.888 |
12 |
16.968 |
Va |
30.047 |
|
3 |
4.242 |
У4 |
17.321 |
y2 |
30.401 |
|
‘/4 |
4.575 |
У2 |
17.675 |
3/4 |
30.754 |
|
‘/2 |
4.949 |
% |
18.028 |
22 |
31.108 |
|
3A |
5.302 |
13 |
18.382 |
У, |
31.461 |
|
4 |
5.656 |
У4 |
18.735 |
y2 |
31.815 |
|
‘/4 |
6.009 |
y2 |
19.089 |
3/4 |
32.168 |
|
y2 |
6.363 |
% |
19.442 |
23 |
32.522 |
|
3A |
6.716 |
14 |
19.796 |
y4 |
32.875 |
|
5 |
7.070 |
y4 |
20.149 |
Уа |
33.229 |
|
У. |
7.423 |
y2 |
20.503 |
3/4 |
33.582 |
|
y2 |
7.777 |
% |
20.856 |
24 |
33.936 |
|
3A |
8.130 |
15 |
21.210 |
y4 |
34.289 |
|
6 |
8.484 |
‘/4 |
21.563 |
y2 |
34.643 |
|
У. |
8.837 |
y2 |
21.917 |
3/4 |
34.996 |
|
y2 |
9.191 |
% |
22.270 |
25 |
35.350 |
|
3/> |
9.544 |
16 |
22.624 |
У4 |
35.703 |
|
7 |
9.898 |
•Л |
22.977 |
y2 |
36.057 |
|
У. |
10.251 |
Vz |
23.331 |
% |
36.410 |
|
Vz |
10.605 |
% |
23.684 |
26 |
36.764 |
|
% |
10.958 |
17 |
24.038 |
У4 |
37.117 |
|
8 |
11.312 |
V4 |
24.391 |
y2 |
37.471 |
|
‘/4 |
11.665 |
У2 |
24.745 |
% |
37.824 |
|
‘/2 |
12.019 |
3/4 |
25.098 |
27 |
38.178 |
|
% |
12.372 |
18 |
25.452 |
1/4 |
38.531 |
|
9 |
12.726 |
‘/4 |
25.805 |
y2 |
38.885 |
|
y4 |
13.079 |
y2 |
26.159 |
3/4 |
39.238 |
|
‘/2 |
13.433 |
% |
26.512 |
28 |
39.592 |
|
3/4 |
13.786 |
19 |
26.866 |
У4 |
39.945 |
|
10 |
14.140 |
% |
27.219 |
y2 |
40.299 |
|
‘/4 |
14.493 |
y2 |
27.573 |
3/4 |
40.652 |
|
‘/2 |
14.847 |
3/4 |
27.926 |
29 |
41.006 |
|
% |
15.200 |
20 |
28.280 |
У4 |
41.359 |
|
11 |
15.554 |
У4 |
28.635 |
y2 |
41.713 |
FIGURE 2.2 ■ Set and travel relationships in inches for 45° offsets.
