Category HIGHWAY ENGINEERING HANDBOOK

Composite pavement deterioration is exhibited in a combination of some flexible pave­ment distresses and some rigid pavement distresses. The most prominent composite pavement distresses, which were defined under flexible or rigid pavement, are raveling, bleeding, rutting, corrugations, pumping, and various slab distresses.

3.8.2 Investigative Methods

If a pavement exhibits structural distresses, such as cracking, patching, potholes, faulting, etc., further evaluation may be necessary to identify the cause of the distress, the extent,

and the strength of the existing pavement system and subgrade. Roadways with high traffic volumes, especially those with high truck volumes, should also be evaluated prior to rehabilitation.

Pavement Coring. Without question, the simplest and most reliable method of identifying pavement deterioration is pavement coring. Pavement coring can be used to investigate many different pavement distress factors, from rigid joint deterioration to stripping in asphalt concrete pavement layers. The following are examples of pavement cores taken in various investigations.

Figure 3.41 shows a core of a composite pavement taken at a transverse joint. The core reveals a tight joint with aggregate interlock and little or no deterioration. The asphalt overlay is left intact. However, during the coring operation, the asphalt portion of the core should be inspected for delaminations between paving layers, rutting of any layers, or stripping of the asphalt from the aggregate.

Figure 3.42 shows a core hole in the pavement taken at a midpanel transverse crack. A wealth of information can be obtained by inspection of the core hole. The core hole reveals aggregate interlock to be questionable. A close inspection revealed the reinforcing mesh to be rusted and broken, not cut by the coring operation. Because the core hole indi­cates most of the aggregate interlock is lost, this crack can be considered a working crack and should be repaired.

Figures 3.43 and 3.44 show the remains of cores taken at transverse joints. It is obvious that these joints need a full-depth repair. Cores should also be taken away from the joint to determine required width of repair.

Figure 3.45 shows a core of an asphalt pavement that indicates a delamination approximately 3 in (75 mm) from the surface. Several cores should be taken to verify the extent of the flaw. A delamination found in an asphalt pavement such as this could result in debonding of the surface layer. If warranted, asphalt milling may be required to a depth sufficient to remove the delamination.

FIGURE 3.42 Hole in pavement after core was drilled at transverse crack in composite pavement.

FIGURE 3.43 Crumbled core taken from transverse joint in rigid pavement.

FIGURE 3.44 Crumbled core taken from transverse joint in composite pavement.

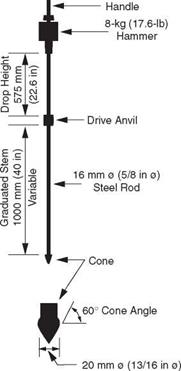
Dynamic Cone Penetrometer. The dynamic cone penetrometer (DCP) is commonly used to determine the stiffness of the base and/or subgrade. As shown in Fig. 3.46, the DCP consists of a 13/16-in (21-mm) diameter, 60° cone mounted on a 5/8-in (16-mm) rod. A 17.6-lb (8-kg) weight is attached to the top of the DCP in such a manner that it can be raised 22.6 in (574 mm) and released while the cone is resting on the base or subgrade.

FIGURE 3.45 Core from flexible pavement indicating delamination about 3 in (75 mm) from surface.

FIGURE 3.46 Schematic of dynamic cone penetrometer. (From Pavement Technology Advisory 97-7, Dynamic Cone Penetrometer, Illinois Department of Transportation, Bureau of Materials and Physical Research, 1997, with permission)

The penetration per drop of the weight is correlated to the CBR or modulus of the base or subgrade. Figure 3.47 shows one such correlation used by the Illinois Department of Transportation. The DCP is popular because it is a relatively inexpensive device that can rapidly determine the stiffness of a base or subgrade in the field.

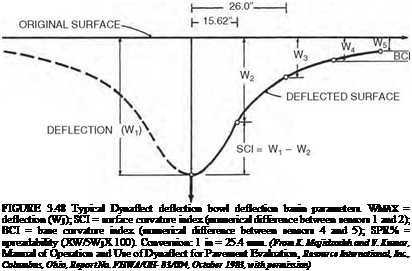
Nondestructive Testing. The most common of the nondestructive testing (NDT) methods is a deflection measuring device such as the falling weight deflectometer (FWD), road rater, and Dynaflect. These devices place a load, either impulse or cyclic, on the pavement and measure the deflection of the pavement using three or more geophones placed at various dis­tances from the load. The measured deflection at each sensor can be described as a “deflection bowl” (see Fig. 3.48). The deflection bowl can be used to evaluate the pavement and to determine the stiffness of the pavement system and individual pavement layers. Stiffness of the layers can be determined using any one of numerous backcalculation programs such as Modulus, Modcomp, Evercalc, etc.; many of which are available in the public domain. The calculated stiffness can then be used to design a rehabilitation treatment. The various deflec­tion parameters shown in Fig. 3.48 can also be used to evaluate the pavement. Table 3.27

0.1 1 10 Penetration Rate, in/blow

— . 49.03” 37.36”

P

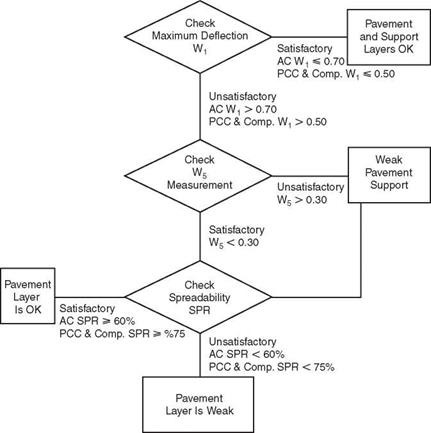
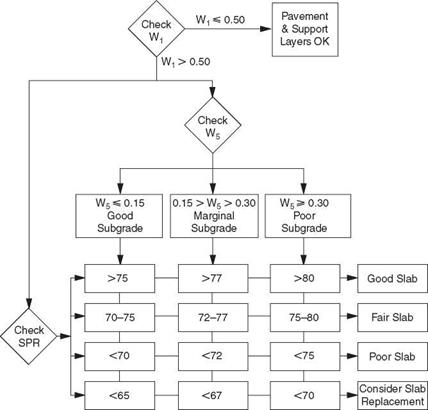
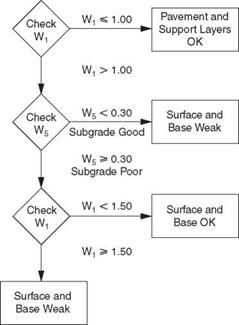
and Figs. 3.49 through 3.52 show an example of how the Dynaflect deflections can be used to determine subgrade and pavement conditions.

Ground Penetrating Radar. Improved analysis software has moved the ground pene­trating radar (GPR) from research to common usage. This GPR consists of a transmitter

TABLE 3.27 Joint Evaluation of Rigid and Composite Pavements Dynaflect SCI (W1 – W2) Joint condition < 0.05 Probably frozen 0.05-0.11 Good load transfer 0.11-0.23 Fair load transfer > 0.23 Poor load transfer Source: K. Majidzadeh and V. Kumar, Manual of Operation and Use of Dynaflect for Pavement Evaluation, Resource International, Inc., Columbus, Ohio, Report No. FHWA/OH-83/004, October 1983, with permission.

FIGURE 3.49 Rigid and flexible (thickness >6 in or 150 mm) pavement evaluation from Dynaflect measurements. (From K. Majidzadeh and V. Kumar, Manual of Operation and Use of Dynaflect for Pavement Evaluation, Resource International, Inc., Columbus, Ohio, Report No. FHWA/OH-83/004, October 1983, with permission)

FIGURE 3.50 Evaluation of rigid and composite pavements from Dynaflect measurements. (From K. Majidzadeh and V. Kumar, Manual of Operation and Use of Dynaflect for Pavement Evaluation, Resource International, Inc., Columbus, Ohio, Report No. FHWA/OH-83/004, October 1983, with permission)

and receiver. The GPR transmits pulses of electromagnetic energy at various frequencies into the pavement system. The pulses are reflected back to the receiver by the interface of the various pavement layers. The dielectric constant of the various pavement layers is determined by coring the pavement and calibrating the GPR. The GPR can be used to determine pavement layer thicknesses, locate voids, and locate areas with high moisture. Use of the GPR has been standardized in ASTM D-4748.

Spectral Analysis of Surface Waves. Spectral analysis of surface waves (SASW) is cur­rently a research tool but may find use as an evaluation tool in the near future. As shown in Fig. 3.53, two or more accelerometers are attached to the pavement in line with the test point. An instrumented hammer is used to generate surface waves. The accelerometers measure the travel time of the surface waves. By varying the distance between two accelerometers or by using multiple accelerometers, data can be gathered for the deeper pavement layers. Analysis of the surface waves can be used to determine the modulus and thickness of each layer in the pavement surface.

FIGURE 3.51 Evaluation of thin (thickness 4 to 6 in) asphalt pavements from Dynaflect measurements. (From K. Majidzadeh and V. Kumar, Manual of Operation and Use of Dynaflect for Pavement Evaluation, Resource International, Inc., Columbus, Ohio, Report No. FHWA/OH-83/004, October 1983, with permission)

Flexible pavement deterioration is exhibited in any combination of the following distresses.

Raveling. Raveling, as shown in Fig. 3.31, is the result of loss of small aggregates from the pavement surface. Raveling can be caused by oxidation of the mix, improper mix design, segregation, or lack of compaction.

Bleeding. Bleeding is the flushing of excess asphalt cement to the surface of the pavement, as evident in Fig. 3.32. Asphalt cement concrete mixtures are more prone to

FIGURE 3.33 Example of small pothole in flexible pavement.

bleed with hotter pavement surface temperatures. Bleeding is a result of excess asphalt cement in the mix and/or low air voids in the mix.

Potholes. One of the most common problems is the development of a pothole (Fig. 3.33). Potholes are small, localized, but deep pavement failures characterized by a round shape. Potholes are caused by weak and wet subbase and/or subgrade. In freeze-thaw environments, potholes are generally formed during the thaw.

Rutting. Rutting (Fig. 3.34) is the longitudinal deformation of the pavement structure within the wheel tracks. Where found only in the uppermost portions of the pavement, it is caused by poor mixture design and lack of stability. Where rutting is deep-seated and found throughout the depth of the pavement structure, it is caused by inadequate pavement structure above the founding layers or by a weak, wet subgrade.

Corrugation. Corrugations (Fig. 3.35) are transverse waves in the pavement profile, which are found most generally at stop lights, at stop signs, or on hills. Corrugations are found in the wheel track and are the result of acceleration and deceleration of heavy trucks in a regular pattern on the roadway surface. The stability of the asphalt mix can also be a contributing factor.

Longitudinal Cracking. Longitudinal cracking, such as shown in Fig. 3.36, is most often found at paving joints established during construction. The construction joint is most gen­erally specified at lane lines. As weathering of the pavement takes place, the longitudinal joint ravels and eventually spalls. Longitudinal cracks found at locations other than paving joints are due to thermal shrinkage from seasonal temperature changes.

Transverse Cracking. As illustrated by Fig. 3.37, transverse cracking is best described by cracks that form across the pavement perpendicular to the centerline.

FIGURE 3.34 Example of rutting in flexible pavement.

Transverse cracking is caused by thermal shrinkage from seasonal temperature changes and age hardening of the binder.

Block Cracking. Block cracking is the combination of longitudinal and transverse cracking, as shown in Fig. 3.38. As the cracks worsen with time as a result of weathering, they join each other and form block cracking.

FIGURE 3.36 Example of longitudinal cracking in flexible pavement.

Wheel Track Cracking. Wheel track cracking is shown in Fig. 3.39. It can be described as mostly longitudinal cracks found at the surface of the pavement within a 3-ft-wide (0.9-m) strip considered to be the wheel track. Wheel track cracking ranges from a single longitudinal crack to a series of interconnected longitudinal cracks, also referred to as alligator cracking. Wheel track cracking is commonly considered to be the most alarming distress found in a flexible pavement. This type of cracking starts at the bottom of the pavement structure and is transmitted to the surface. By the time alligator cracking can be detected by visual inspection, the pavement is generally considered to be failed.

FIGURE 3.38 Example of block cracking in flexible pavement.

FIGURE 3.40 Example of edge cracking in flexible pavement.

Edge Cracking. Edge cracking, as shown in Fig. 3.40, is a series of short longitudinal or irregular-shaped cracks at the outer 15 in (380 mm) of the pavement. Edge cracking is a result of lack of support outside the pavement edge.

Continuously reinforced concrete pavement deterioration is exhibited by the same dis­tresses discussed for jointed concrete pavement along with the following additional considerations.

Settlement. As previously stated, settlement as displayed by a depression in the pro­file of the pavement affects the smoothness of a pavement. It may be the result of poor construction practice such as poor compaction over a utility, poor grade control during final grading of the subgrade, or localized soil conditions that cannot resist additional overburden or increased loading. Repair methods consist of replacement to the cor­rected profile or an overlay. However, settlements are more predominant in CRC pavement, because transverse cracks are inherently more numerous. With the trans­verse cracking at a spacing of 5 to 8 ft (1.5 to 2.4 m), the pavement is able to bend more freely and does not bridge weak foundations as effectively.

FIGURE 3.28 Example of corner break in jointed rigid pavement.

Trans-verse Cracking. Although CRC pavement is designed to have transverse

cracks, the cracks should be spaced properly. Transverse cracks spaced too closely (less than 3 ft (0.9 m), as illustrated in Fig. 3.29) have a good chance of interconnect­ing, because they do not form uniformly straight and perpendicular to the centerline. Thus, as they interconnect, spalling will occur and pavement failures will result. On the other hand, transverse cracks spaced too far apart create higher stresses than the reinforcement can tolerate, and this can also result in pavement failures. Although

FIGURE 3.30 Example of punchout in continuously reinforced rigid pavement.

incorrect transverse crack spacing is not a distress by itself, it must be monitored to help pavement engineers predict failures. Once failures are evident, they must be repaired by full-depth pavement removal and replacement. It is important to reestablish conti­nuity of the reinforcement within the repair.

Punchouts. Figure 3.30 shows a punchout in a CRC pavement. A punchout is formed by the combination of intersecting transverse and longitudinal cracks over an area of weak foundation.

Jointed rigid pavement deterioration is exhibited in any combination of the following distresses:

Surface Deterioration. Surface deterioration (Fig. 3.22) is the result of loss of cement at the surface of the slab (scaling). It is generally caused by excessive surface water and finishing practice, or the loss of both small aggregates and cement caused by abrasion from tires. Surface deterioration affects the noise level of a pavement and cannot be repaired. Surface deterioration by itself is generally of little concern.

TABLE 3.26 Pavement Coefficients for Flexible Section Design, Louisiana Strength* Coefficient I. Surface course* Asphaltic concrete Types 1, 2, and 4 BC and WC 1000+ 0.40 Types 3 WC 1800+ 0.44 BC 1500+ 0.43 II. Base course Untreated* Sand clay gravel—grade A 3.3— 0.08 Sand clay gravel—grade B 3.5 — 0.07 Shell and sand-shell 2.2— 0.10 Cement-treated§ Soil-cement 300+ 0.15 Sand clay gravel—grade B 500+ 0.18 Shell and sand-shell 500+ 0.18 Shell and sand-shell 650+ 0.23 Lime-treated* Sand-shell 2.0— 0.12 Sand clay gravel—grade B 2.0— 0.12 Asphalt treated* Hot-mix base course (type 5A) 1200+ 0.34 Hot-mix base course (type 5B) 800+ 0.30 III. Subbase course* Lime-treated sand clay gravel—grade B 2.0— 0.14 Shell and sand-shell 2.0— 0.14 Sand clay gravel—grade B 3.5 — 0.11 Lime-treated soil 3.5 — 0.11 Old gravel or shell roadbed (8-in thickness) (200 mm) — 0.11 Sand (R-value) 55+ 0.11 Suitable material—A—6 (PI = 15—) — 0.04 IV. Coefficients for bituminous concrete overlay Base course Bituminous concrete pavement New 0.40 Old 0.24 Portland cement concrete pavement New 0.50 Old, fair condition 0.40 Old, failed 0.20 Old, pumping 0.10 Old, pumping (to be undersealed) 0.35 *Refer to the following footnotes for strength designations. See the AASHTO guide referenced below for further details. ^Marshall stability number. *Texas triaxial values. §Compressive strength, lb/in2 (1 lb/in2 = 6.895 X 10—3 MPa) Source: Interim Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1972 (rev. 1981), with permission.

FIGURE 3.21 Procedure for determining thicknesses of layers using a layered analysis approach. a, D, m, and SN are defined in the text and are minimum required values. An asterisk indicates that the value actually used is represented; this value must be equal to or greater than the required value. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

Popouts. Figure 3.23 shows a typical popout. Popouts are generally due to high steel placement, but also may be the result of poor-quality aggregate, which disintegrates, causing cavities at the surface of the slab. Popouts affect the noise level of a pavement and cannot be repaired. Popouts by themselves are generally of little concern.

Pumping. Pumping is defined as the ejection of subbase or subgrade materials from under a pavement through a joint or crack and out onto the pavement and shoulder. The loss of subbase or subgrade material causes loss of support and leads to corner breaks and faulting. The existence of pumping can be determined visually by the pres­ence of soil stains at the joints or cracks on the adjacent shoulder.

Faulting. Faulting is a result of the loss of load transfer across a joint or crack, which causes the slab on one side of the joint or crack to be at a lower elevation than the slab on the other side. Faulting (Fig. 3.24) is generally a result of pumping. Faulting affects the noise level and the smoothness of a pavement. It is generally con­sidered excessive when faulting exceeds 1/4 in (6 mm). Faulting can be corrected by pavement grinding, joint or crack repair, or slab jacking. However, unless load transfer

FIGURE 3.22 Example of surface deterioration in jointed rigid pavement.

FIGURE 3.24 Example of faulting at joint in rigid pavement; pavement on right is about 1/2 in (13 mm) lower than that on left.

is established across the joint or crack and any existing voids under the joint or crack are filled, faulting can be expected to return.

Settlement. Settlement is the result of poor construction practice. It may be due to either poor compaction over a utility, poor grade control during the final grading of the subgrade, or possible localized soil conditions that cannot resist additional over­burden or increased loading. Settlement, which is displayed by a depression in the profile of the pavement, affects smoothness. Repair methods consist of replacement to the corrected profile, or an overlay of some type. Settlements are generally of little concern unless they are numerous and severely affect the ride of the pavement.

Joint Spalling. Figure 3.25 shows typical joint spalling, defined as deterioration of the concrete slab around transverse or longitudinal joints. The deterioration is generally only to partial depth and is visible from the surface of the slab. Joint spalling may result from poor-quality aggregates (D cracking); improperly placed dowels, tie-bars, or dowel baskets; or excessive expansion of the concrete (pressure). Repair of spalled joints can be accomplished by either partial-depth joint repairs or full-depth joint repairs.

FIGURE 3.25 Example of joint spalling in rigid pavement.

Trans-verse Cracking. A significant transverse crack is depicted in Fig. 3.26.

Transverse cracking severity varies from hairline cracks to cracks sufficiently wide to completely separate the slab into two distinct pieces. Hairline cracks are expected in reinforced concrete and pose no expected problems. In plain concrete pavement, a hairline crack can be a sign of future problems. Without reinforcing mesh to hold the crack together, the long-term performance of the slab is questionable; however, as long as the crack is tightly closed (hairline), it poses no problem. Regardless of

FIGURE 3.27 Example of longitudinal crack in jointed rigid pavement.

whether the pavement contains mesh, cracks that have separated by a distance greater than one-half of the largest aggregate diameter are generally considered to be failed.

Longitudinal Cracking. Longitudinal cracking, such as shown in Fig. 3.27, may be caused by excessive lane widths, longitudinal joints that were not sawed properly, or local conditions that increase the stress level along the pavement. Longitudinal cracking is pri­marily a concern when it occurs within the wheel track. Where a longitudinal crack is faulted, spalled, pumping, or working and is in the wheel path, it can become a safety hazard.

Corner Breaks. As illustrated in Fig. 3.28, corner breaks are cracks found at the cor­ner of the slab. They usually propagate from the transverse joint to the longitudinal joint. Corner breaks are full-depth cracks and are generally the result of loss of sup­port under the corner of the slab.

Pavement deterioration or distress can be classified into two basic categories for all pave­ment types—structural and functional. The most serious category is structural. Structural deterioration results in reduced ability to carry load and a decreased pavement life. Functional deterioration can lead to and accelerate structural deterioration, but it is only related to ride quality and frictional characteristics. A third type is environmental deterioration, which is a form of material-related distress. Environmental deterioration affects pavement materials and will generally exhibit itself as either functional or structural deterioration.

log10 MR – 8.07

+ 2.32

S0 = 0.35 MR = 5000 lb/in2 Д (lb/in2) 1.9 Solution: SN = 5.0

FIGURE 3.17 Design chart for flexible pavements based on using mean values for each variable. Conversions: 1 kip = 4448 N, 1 lb/in2 = 6.895 X 10-3 MPa, 1 kip/in2 = 6.895 MPa. {From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

TABLE 3.25 Recommended m Values for Modifying Structural Layer Coefficients of Untreated Base and Subbase Materials in Flexible Pavements Quality of drainage Percent of time pavement structure is exposed to moisture levels approaching saturation Less than 1% 1-5% 5-25% Greater than 25% Excellent 1.40-1.35 1.35-1.30 1.30-1.20 1.20 Good 1.35-1.25 1.25-1.15 1.15-1.00 1.00 Fair 1.25-1.15 1.15-1.05 1.00-0.80 0.80 Poor 1.15-1.05 1.05-0.80 0.80-0.60 0.60 Very poor 1.05-0.95 0.95-0.75 0.75-0.40 0.40 Source: Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission.

FIGURE 3.18 Chart for estimating structural layer coefficient (a^ of dense-graded asphalt concrete based on the resilient modulus. Conversion: 1 lb/in2 = 6.895 X 10_3 MPa. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

0.20 0.18 0.16 0.14 0.12 0.10 0.08 0.06 0.04

40 –

(1) Scale derived by averaging correlations obtained from Illinois.

(2) Scale derived by averaging correlations obtained from California, New Mexico, and Wyoming.

(31 Scale derived by averaging correlations obtained from Texas

(4) Scale derived on NCHRP project.

FIGURE 3.19 Variation in granular base layer coefficient (a2) with various base strength parameters. Conversion: 1 lb/in2 = 6.895 X 10~3 MPa. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

Pavement deterioration is an important measurement for a pavement engineer. To determine the remaining life of a pavement, or the amount of pavement repair required to extend a pavement life for a given time period, or the most appropriate time for pavement repair, the amount and type of deterioration in a pavement must be measured. Methods of measurement of pavement deterioration vary, but most are similar in that they all require a visual inspection of the pavement and a somewhat subjective distress rating.

FIGURE 3.20 Variation in granular base layer coefficient (a3) with various subbase strength parameters. Conversion: 1 lb/in2 = 6.895 X 10_3 MPa. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

Project-level pavement management is responsible for continuous evaluation of pave­ment’s present serviceability, monitoring of the pavement loading rate, determination of the cause and rate of pavement deterioration, prediction of optimal time for inter­vention, and evaluation of the most economical rehabilitation strategy.

Pavement management can be applied at the project level or at the network level. Although both levels are very dependent upon one another, they are seldom applied for the same purpose. The network level applies to the whole system in a global sense. Network refers to systemwide averages and is used for system budgeting and performance modeling. This chapter addresses only the project-level aspects. Project-level pavement management is considered to be more complicated and more important than pavement design. Pavement management is applied throughout the life of a pavement, whereas pavement design is com­pleted and forgotten once the pavement is initially in service.

Д (Ib/in2) 4.5- 1.5

1.624* 107

18.42 (Ec/k)02

s’c* cd (d0-75- і. ізг|

+ (4.22- O.32pt)*log10

215.63 *J P0J5-

Д (Ib/in2) = 4.2-2.5= 1.7 W18 = 5.1 x 106 (18-kip ESAL) Solution: D = 10.0 in.

(nearest half-inch, from part b )

FIGURE 3.15 Design chart for rigid pavements based on using mean values for each input variable. Conversions: 1 lb/in2 = 6.895 X 10-3 MPa, 1 lb/in3 = 271.4 X 10-4 N/mm2, 1 in = 25.4 mm. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

0­

10­

Note:

005

Relative Damage

Month

Jan.

Feb

Mar.

May

June

July

Sept

Oct.

Nov.

Dec

10.0

Summation: Eu

13.0

Average: u

Effective Roadbed Soil Resilient Modulus, M„ (Ib/in >

FIGURE 3.16 Chart for estimating effective roadbed soil resilient modulus for flexible pavements designed using the serviceability criteria. Conversion: 1 lb/in2 = 6.895 X 10_3 MPa. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

Determine Pavement Structural Number. The flexible pavement process involves the calculation of a pavement structural number. This is an abstract number reflecting the relative strength contribution of all layers in the pavement buildup. The structural number SN is calculated using the design values determined as outlined above in the nomograph shown in Fig. 3.17. The design thickness for each layer is determined to satisfy the following equation:

SN = a1D1 + a2D2m2 + a3D3m3

where a1, a2, a3 = structural coefficients of surface, base, and subbase, respectively

D1, D2, D3 = thickness of surface, base, and subbase, respectively, in m2, m3 = drainage coefficients for base and subbase (see Table 3.25)

The structural coefficients of the asphalt layer, granular base, and subbase can be esti­mated using Figs. 3.18, 3.19, and 3.20, respectively, or can be estimated from Table 3.26.

Select Layer Material and Thickness. Once the structural coefficients are known, the thickness of the individual layers is determined by varying D1, D2, and D3 until the calcu­lated SN is equal to or greater than the required SN. Unbound bases are commonly speci­fied to the nearest 1 in (25 mm), and asphalt concrete is normally specified to the nearest 1/4 in (6 mm). The procedure shown in Fig. 3.21 illustrates one method recommended for determining layer thickness. This procedure designs the upper layers to protect the lower layers.

3.

Determine the drainage coefficient for the pavement.

4. Select the design serviceability loss.

5. Estimate the total number of 18-kip (80-kN) equivalent single-axle loads for the design period.

6. Select a level of reliability and the overall standard deviation.

7. Determine slab thickness and steel reinforcement.

Determine Effective Subgrade Modulus. The first step in designing the thickness of a rigid pavement is the determination of the effective modulus of subgrade reaction. The effective modulus (or composite modulus) is the modulus of subgrade reaction after cor­rection for use of subbase, seasonal variation in subgrade and subbase strength, rigid foundation within 10 ft (3 m) of the surface, and loss of support. Figure 3.11 is used to determine the composite modulus of subgrade reaction when a subbase will be used under the concrete pavement. If the pavement will be placed directly on the subgrade, the AASHTO Pavement Design Guide recommends a composite modulus of subgrade reaction of:

where k is in lb/in3 and MR is in lb/in2.

When a stiff layer (bedrock, etc.) is located within 10 ft (3 m) of the surface, the stiff layer will provide additional support for the pavement. Figure 3.12 is used to correct the composite modulus of subgrade reaction for this additional support.

Example:

DSB = 6 in (150 mm)

ESB = 20,000 lb/in2 (138 MPa)

MR = 7,000 lb/in2 (48 MPa)

Solution: k* = 400 lb/in3 (0.109 N/mm3)

2000 3000 5000

7000

10,000

12,000

16,000

20,000

FIGURE 3.11 Chart for estimating composite modulus of subgrade reaction kra, assuming a semi­infinite subgrade depth; defined as over 10 ft (3 m) below subgrade surface. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

In regions where large moisture variations, freeze and thaw, etc., will affect the strength of the subgrade soils and subgrade, AASHTO provides a procedure to modify the composite modulus of subgrade reaction. Table 3.22 provides a worksheet, and Table 3.23 shows an example. The seasonal variation in strength is determined using laboratory procedures or nondestructive testing (NDT). The seasonal strength of the subbase and subgrade is entered in columns 2 and 3 of Table 3.22. The composite modulus of subgrade reaction is determined using Fig. 3.11 and entered in column 4. If a rigid foundation is present within 10 ft (3 m) of the surface, the k value is corrected

Example:

MR = 4000 Ib/in2 (27.6 MPa)

□SG = 5 ft (1.5 m)

= 230 Ib/in3 (0.062 N/mm3)

Solution: к = 300 Ib/in3 (0.081 N/mm3)

Modulus of Subgrade Reaction, к (ib/in3)

(modified lo account for presence of rigid foundation near surface)

FIGURE 3.12 Chart for modifying modulus of subgrade reaction to consider effect of rigid foundation near the surface. Conversions: 1 lb/in2 = 6.895 X 10-3 MPa, 1 lb/in3 = 271.4 X 10-4 N/mm2, 1 ft = 0.305 m. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

Trial subbase: Type _________________ Depth to rigid foundation, ft

Thickness, in___________ Projected slab thickness, in

Loss of support, LS______

Month (1)	Roadbed modulus Mr, lb/in2 (2)	Subbase modulus, ESb, lb/in2 (3)	Composite k value, lb/in3 (Fig. 3.11) (4)	k value, lb/in3 on rigid foundation (Fig. 3.12) (5)	Relative damage u (Fig. 3.13) (6)
January
February
March
April
May
June
July
August
September
October
November
December

Average: ur = ____ n Summation: =

Effective modulus of subgrade reaction k (lb/in3) =_________

Corrected for loss of support (Fig. 3.14): k (lb/in3) =________

Conversions: 1 lb/in2 = 6.895 X 10_3 MPa, 1 lb/in3 = 271.4 X 10_4N/mm2, 1 in = 25.4 mm, 1 ft = 0.305 m. Source: Guide for Design of Pavement Structures, American Association of State Highway and

Transportation Officials, Washington, D. C., 1993, with permission.

TABLE 3.23 Example Application of Method for Estimating Effective Modulus of Subgrade Reaction

Trial subbase: Type ____ Grammar_____ Depth to rigid foundations, ft__________ 5

Thickness, in ___________ Projected slab thickness, in ___________ 99

Loss of support, LS І-0

Month (1)	Roadbed modulus Mr, lb/in2 (2)	Subbase modulus, ESB, lb/in2 (3)	Composite k value, lb/in3 (Fig – 3-11) (4)	k value, lb/in3 on rigid foundation (Fig – 3,12) (5)	Relative damage ur (Fig – 3-13) (6)
January	20,000	50,000	1,100	1,350	0-35
February	20,000	50,000	1,100	1,350	0-35
March	2,500	15,000	160	230	0-86
April	4,000	15,000	230	300	0-78
May	4,000	15,000	230	300	0-78
June	7,000	20,000	410	540	0-60
July	7,000	20,000	410	540	0-60
August	7,000	20,000	410	540	0-60
September	7,000	20,000	410	540	0-60
October	7,000	20,000	410	540	0-60
November	4,000	15,000	230	300	0-78
December	20,000	50,000	1,100	1,350	0-35

Summation: Xu = 7-25

Average: йг = X r =____ °-60________ r n

Effective modulus of subgrade reaction k (lb/in3) = 540 Corrected for loss of support (Fig – 3-14): k (lb/in3) = 170

Conversions: See Table 3-22-

Source: Guide for Design of Pavement Structures, American Association of State Highway and

Transportation Officials, Washington, D-C-, 1993, with permission-

FIGURE 3.13 Chart for estimating relative damage to rigid pavements based on slab thickness and underlying support. Conversions: 1 lb/in3 = 271.4 X 10_4 N/mm2, 1 in = 25.4 m. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

using Fig. 3.12 and entered in column 5. The corrected k value is then used in Fig. 3.13 to determine the seasonal or relative damage factor, which is entered in column 6. The sum of relative damage is divided by the total number of periods to determine the average rel­ative damage factor. This value is entered in Fig. 3.13 to determine the average composite modulus of subgrade reaction for the year. Many concrete pavements fail as a result of pumping or loss of support under the slab. Figure 3.14 is provided to correct the effective modulus of subgrade reaction for loss of support. This figure lowers the k so that the

FIGURE 3.14 Chart for correction of effective modulus of subgrade reaction for potential loss of subbase support. Conversion: 1 lb/in3 = 271.4 X 10~4 N/mm2. (From Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission)

stress in the slab will be the same as for a slab with a void. Although the AASHTO procedure includes design for loss of support, it is recommended that a pavement base be designed to prevent or reduce loss of support, especially under pavements supporting a large number of heavy loads in wet areas. The cost of providing a base to resist loss of support may be less than the cost of restoring support in the future.

Select Pavement Material Properties. The next step in the design of a rigid pavement is to select material properties. The reliability level and overall standard deviation consider the variation in material properties. Therefore, average material property values must be used in design. The concrete material values needed for design are the average concrete modulus of elasticity and the average concrete modulus of rupture. These values are not known until after construction of the pavement unless the plans for the pavement contain a performance specification. Material properties from past pave­ment construction may be used for design purposes provided a similar mix will be used. American Society for Testing and Materials’ “Test Method for Static Modulus of Elasticity and Poisson’s Ratio of Concrete in Compression,” ASTM C469, details the laboratory test method for determining the concrete modulus of elasticity. ASTM C78,

TABLE 3.24 Recommended Values of Drainage Coefficient Cd for Rigid Pavement Design Quality of drainage Percent of time pavement structure is exposed to moisture levels approaching saturation Less than 1% 1-5% 5-25% Greater than 25% Excellent 1.25-1.20 1.20-1.15 1.15-1.10 1.10 Good 1.20-1.15 1.15-1.10 1.10-1.00 1.00 Fair 1.15-1.10 1.10-1.00 1.00-0.90 0.90 Poor 1.10-1.00 1.00-0.90 0.90-0.80 0.80 Very poor 1.00-0.90 0.90-0.80 0.80-0.70 0.70 Source: Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission.

“Test Method for Flexural Strength of Concrete (Using Simple Beam with Third-Point Loading),” details the laboratory test method for determining the concrete flexural strength (modulus of rupture).

Determine Drainage Coefficient. The drainage coefficient is used to modify the design thickness for drainage conditions. Moisture affects the pavement performance by decreasing the strength of the subgrade and subbase material and affects the warping and curling behavior of the concrete slabs. The intent of the drainage coefficient is to allow performance prediction for pavements without a proper drainage system. Increasing the pavement thickness should not be used in lieu of a properly designed drainage system. Recommended values for the drainage coefficient are given in Table 3.24. The Federal Highway Administration’s “Highway Subdrainage Design Manual,” Report No. FHWA-TS-80-224, provides a procedure that may be used to determine drainage times for base material. (See Arts. 5.4.5 and 5.4.6.)

Select Design Serviceability Loss. The design serviceability loss is the amount of serviceability loss the agency will tolerate before rehabilitation. To select a design ser­viceability loss, the designer needs to know the initial serviceability and the terminal serviceability of the pavement. The initial serviceability is the serviceability immedi­ately after construction. Since this value is unknown at the time of construction, the designer will usually use the average initial serviceability of previously constructed pavements. The terminal serviceability is the serviceability of a pavement immediately before rehabilitation. The terminal serviceability is a function of traffic volume and speed. A low-volume road with low speeds may be allowed to deteriorate to a lower serviceability than a high-volume freeway, since the associated user costs will be lower. The terminal serviceability used by an agency is a policy decision. Common terminal serviceabilities are 2.5 for high-volume roads and 2.0 for low-volume roads.

Estimate ESALs. The daily ESAL loadings are determined as outlined in Art. 3.5.2. The total number of ESAL loadings for design is the cumulative number of ESAL loadings expected over the design life of the pavement. This value can be determined by assuming a growth rate or, if the pavement is being built on an existing alignment, by extrapolating past traffic patterns.

Determine Slab Thickness and Reinforcement. The design slab thickness is deter­mined by using the design values as outlined above in the nomograph shown in Fig. 3.15. The design thickness is usually rounded up to the nearest 1/2 or 1 in (13 or 25 mm) depending on the local practice for specifying slab thickness. As mentioned in Art. 3.5.2, if the design thickness varies significantly from the thickness used to determine the equivalency factors, the equivalency factors should be recalculated and the thickness design checked. Determination of steel reinforcement content, if used, is detailed in Art. 3.1.1.

The resilient modulus is a measure of the ability of a soil or granular base to resist permanent deformation under repeated loading. Many soils are stress-dependent. As the stress level increases, these soils will behave in a nonlinear fashion. Fine-grain soils tend to be stress-softening, whereas granular soils tend to be stress-hardening. Laboratory procedures for determining resilient modulus have been published by AASHTO as test method T307, or NCHRP as test method 1-38A. A typical setup for the laboratory test is shown in Fig. 3.9. The stress due to the repeated load applied through the load actuator is the deviator stress and is intended to duplicate the effect of loads passing over a section of pavement. The confining stress within the chamber is intended to duplicate the confinement of the soil within the subgrade. A typical load-response curve is shown in Fig. 3.10. As shown, the resilient modulus (MR) is the ratio of deviator stress to strain in the elastic range.

The laboratory procedures for determining resilient modulus are complex and time­consuming. Many equations have been developed relating the resilient modulus to soil properties that are more easily determined. One such property is the California

TABLE 3.11 Axle Load Equivalency Factors for Rigid Pavements, Tandem Axles, and pt of 2.0 Axle load Slab thickness D, in kips kN 6 7 8 9 10 11 12 13 14 2 9 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 4 18 0.0006 0.0005 0.0005 0.0005 0.0005 0.0005 0.0005 0.0005 0.0005 6 27 0.002 0.002 0.002 0.002 0.002 0.002 0.002 0.002 0.002 8 36 0.006 0.006 0.005 0.005 0.005 0.005 0.005 0.005 0.005 10 44 0.014 0.013 0.013 0.012 0.012 0.012 0.012 0.012 0.012 12 53 0.028 0.026 0.026 0.025 0.025 0.025 0.025 0.025 0.025 14 62 0.051 0.049 0.048 0.047 0.047 0.047 0.047 0.047 0.047 16 71 0.087 0.084 0.082 0.081 0.081 0.080 0.080 0.080 0.080 18 80 0.141 0.136 0.133 0.132 0.131 0.131 0.131 0.131 0.131 20 89 0.216 0.210 0.206 0.204 0.203 0.203 0.203 0.203 0.203 22 98 0.319 0.313 0.307 0.305 0.304 0.303 0.303 0.303 0.303 24 107 0.454 0.449 0.444 0.441 0.440 0.439 0.439 0.439 0.439 26 116 0.629 0.626 0.622 0.620 0.618 0.618 0.618 0.618 0.618 28 125 0.852 0.851 0.850 0.850 0.850 0.849 0.849 0.849 0.849 30 133 1.13 1.13 1.14 1.14 1.14 1.14 1.14 1.14 1.14 32 142 1.48 1.48 1.49 1.50 1.51 1.51 1.51 1.51 1.51 34 151 1.90 1.90 1.93 1.95 1.96 1.97 1.97 1.97 1.97 36 160 2.42 2.41 2.45 2.49 2.51 2.52 2.53 2.53 2.53 38 169 3.04 3.02 3.07 3.13 3.17 3.19 3.20 3.20 3.21 40 178 3.79 3.74 3.80 3.89 3.95 3.98 4.00 4.01 4.01 42 187 4.67 4.59 4.66 4.78 4.87 4.93 4.95 4.97 4.97 44 196 5.72 5.59 5.67 5.82 5.95 6.03 6.07 6.09 6.10 46 205 6.94 6.76 6.83 7.02 7.20 7.31 7.37 7.41 7.43 48 214 8.36 8.12 8.17 8.40 8.63 8.79 8.88 8.93 8.96 50 222 10.00 9.69 9.72 9.98 10.27 10.49 10.62 10.69 10.73 52 231 11.9 11.5 11.5 11.8 12.1 12.4 12.6 12.7 12.8 54 240 14.0 13.5 13.5 13.8 14.2 14.6 14.9 15.0 15.1 56 249 16.5 15.9 15.8 16.1 16.6 17.1 17.4 17.6 17.7 58 258 19.3 18.5 18.4 18.7 19.3 19.8 20.3 20.5 20.7 60 267 22.4 21.5 21.3 21.6 22.3 22.9 23.5 23.8 24.0 62 276 25.9 24.9 24.6 24.9 25.6 26.4 27.0 27.5 27.7 64 285 29.9 28.6 28.2 28.5 29.3 30.2 31.0 31.6 31.9 66 294 34.3 32.8 32.3 32.6 33.4 34.4 35.4 36.1 36.5 68 302 39.2 37.5 36.8 37.1 37.9 39.1 40.2 41.1 41.6 70 311 44.6 42.7 41.9 42.1 42.9 44.2 45.5 46.6 47.3 72 320 50.6 48.4 47.5 47.6 48.5 49.9 51.4 52.6 53.5 74 329 57.3 54.7 53.6 53.6 54.6 56.1 57.7 59.2 60.3 76 338 64.6 61.7 60.4 60.3 61.2 62.8 64.7 66.4 67.7 78 347 72.5 69.3 67.8 67.7 68.6 70.2 72.3 74.3 75.8 80 356 81.3 77.6 75.9 75.7 76.6 78.3 80.6 82.8 84.7 82 365 90.9 86.7 84.7 84.4 85.3 87.1 89.6 92.1 94.2 84 374 101. 97. 94. 94. 95. 97. 99. 102. 105. 86 383 113. 107. 105. 104. 105. 107. 110. 113. 116. 88 391 125. 119. 116. 116. 116. 118. 121. 125. 128. 90 400 138. 132. 129. 128. 129. 131. 134. 137. 141. Conversion: 1 in = 25.4 mm.

Axle load				Slab thickness D, in
kips	kN	6	7	8	9	10	11	12	13	14
2	9	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001
4	18	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003
6	27	0.0010	0.0009	0.0009	0.0009	0.0009	0.0009	0.0009	0.0009	0.0009
8	36	0.002	0.002	0.002	0.002	0.002	0.002	0.002	0.002	0.002
10	44	0.005	0.005	0.005	0.005	0.005	0.005	0.005	0.005	0.005
12	53	0.010	0.010	0.009	0.009	0.009	0.009	0.009	0.009	0.009
14	62	0.018	0.017	0.017	0.016	0.016	0.016	0.016	0.016	0.016
16	71	0.030	0.029	0.028	0.027	0.027	0.027	0.027	0.027	0.027
18	80	0.047	0.045	0.044	0.044	0.043	0.043	0.043	0.043	0.043
20	89	0.072	0.069	0.067	0.066	0.066	0.066	0.066	0.066	0.066
22	98	0.105	0.101	0.099	0.098	0.097	0.097	0.097	0.097	0.097
24	107	0.149	0.144	0.141	0.139	0.139	0.138	0.138	0.138	0.138
26	116	0.205	0.199	0.195	0.194	0.193	0.192	0.192	0.192	0.192
28	125	0.276	0.270	0.265	0.263	0.262	0.262	0.262	0.262	0.261
30	133	0.364	0.359	0.354	0.351	0.350	0.349	0.349	0.349	0.349
32	142	0.472	0.468	0.463	0.460	0.459	0.458	0.458	0.458	0.458
34	151	0.603	0.600	0.596	0.594	0.593	0.592	0.592	0.592	0.592
36	160	0.759	0.758	0.757	0.756	0.755	0.755	0.755	0.755	0.755
38	169	0.946	0.947	0.949	0.950	0.951	0.951	0.951	0.951	0.951
40	178	1.17	1.17	1.18	1.18	1.18	1.18	1.18	1.18	1.19
42	187	1.42	1.43	1.44	1.45	1.46	1.46	1.46	1.46	1.46
44	196	1.73	1.73	1.75	1.77	1.78	1.78	1.79	1.79	1.79
46	205	2.08	2.07	2.10	2.13	2.15	2.16	2.16	2.16	2.17
48	214	2.48	2.47	2.51	2.55	2.58	2.59	2.60	2.60	2.61
50	222	2.95	2.92	2.97	3.03	3.07	3.09	3.10	3.11	3.11
52	231	3.48	3.44	3.50	3.58	3.63	3.66	3.68	3.69	3.69
54	240	4.09	4.03	4.09	4.20	4.27	4.31	4.33	4.35	4.35
56	249	4.78	4.69	4.76	4.89	4.99	5.05	5.08	5.09	5.10
58	258	5.57	5.44	5.51	5.66	5.79	5.87	5.91	5.94	5.95
60	267	6.45	6.29	6.35	6.53	6.69	6.79	6.85	6.88	6.90
62	276	7.43	7.23	7.28	7.49	7.69	7.82	7.90	7.94	7.97
64	285	8.54	8.28	8.32	8.55	8.80	8.97	9.07	9.13	9.16
66	294	9.76	9.46	9.48	9.73	10.02	10.24	10.37	10.44	10.48
68	302	11.1	10.8	10.8	11.0	11.4	11.6	11.8	11.9	12.0
70	311	12.6	12.2	12.2	12.5	12.8	13.2	13.4	13.5	13.6
72	320	14.3	13.8	13.7	14.0	14.5	14.9	15.1	15.3	15.4
74	329	16.1	15.5	15.4	15.7	16.2	16.7	17.0	17.2	17.3
76	338	18.2	17.5	17.3	17.6	18.2	18.7	19.1	19.3	19.5
78	347	20.4	19.6	19.4	19.7	20.3	20.9	21.4	21.7	21.8
80	356	22.8	21.9	21.6	21.9	22.6	23.3	23.8	24.2	24.4
82	365	25.4	24.4	24.1	24.4	25.0	25.8	26.5	26.9	27.2
84	374	28.3	27.1	26.7	27.0	27.7	28.6	29.4	29.9	30.2
86	383	31.4	30.1	29.6	29.9	30.7	31.6	32.5	33.1	33.5
88	391	34.8	33.3	32.8	33.0	33.8	34.8	35.8	36.6	37.1
90	400	38.5	36.8	36.2	36.4	37.2	38.3	39.4	40.3	40.9

Conversion: 1 in = 25.4 mm.

TABLE 3.13 Axle Load Equivalency Factors for Rigid Pavements, Single Axles, and pt of 2.5 Axle load Slab thickness D, in kips kN 6 7 8 9 10 11 12 13 14 2 9 0.0002 0.0002 0.0002 0.0002 0.0002 0.0002 0.0002 0.0002 0.0002 4 18 0.003 0.002 0.002 0.002 0.002 0.002 0.002 0.002 0.002 6 27 0.012 0.011 0.010 0.010 0.010 0.010 0.010 0.010 0.010 8 36 0.039 0.035 0.033 0.032 0.032 0.032 0.032 0.032 0.032 10 44 0.097 0.089 0.084 0.082 0.081 0.080 0.080 0.080 0.080 12 53 0.203 0.189 0.181 0.176 0.175 0.174 0.174 0.173 0.173 14 62 0.376 0.360 0.347 0.341 0.338 0.337 0.336 0.336 0.336 16 71 0.634 0.623 0.610 0.604 0.601 0.599 0.599 0.599 0.598 18 80 1.00 1.00 1.00 1.00 1.00 1.00 1.00 1.00 1.00 20 89 1.51 1.52 1.55 1.57 1.58 1.58 1.59 1.59 1.59 22 98 2.21 2.20 2.28 2.34 2.38 2.40 2.41 2.41 2.41 24 107 3.16 3.10 3.22 3.36 3.45 3.50 3.53 3.54 3.55 26 116 4.41 4.26 4.42 4.67 4.85 4.95 5.01 5.04 5.05 28 125 6.05 5.76 5.92 6.29 6.61 6.81 6.92 6.98 7.01 30 133 8.16 7.67 7.79 8.28 8.79 9.14 9.35 9.46 9.52 32 142 10.8 10.1 10.1 10.7 11.4 12.0 12.3 12.6 12.7 34 151 14.1 13.0 12.9 13.6 14.6 15.4 16.0 16.4 16.5 36 160 18.2 16.7 16.4 17.1 18.3 19.5 20.4 21.0 21.3 38 169 23.1 21.1 20.6 21.3 22.7 24.3 25.6 26.4 27.0 40 178 29.1 26.5 25.7 26.3 27.9 29.9 31.6 32.9 33.7 42 187 36.2 32.9 31.7 32.2 34.0 36.3 38.7 40.4 41.6 44 196 44.6 40.4 38.8 39.2 41.0 43.8 46.7 49.1 50.8 46 205 54.5 49.3 47.1 47.3 49.2 52.3 55.9 59.0 61.4 48 214 66.1 59.7 56.9 56.8 58.7 62.1 66.3 70.3 73.4 50 222 79.4 71.7 68.2 67.8 69.6 73.3 78.1 83.0 87.1 Conversion: 1 in = 25.4 mm. Source: Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission.

Bearing Ratio (CBR). Common equations using CBR to calculate resilient modulus values include the following:

E (lb/in2) = 1500 CBR (Shell Oil Co.)

E (lb/in2) = 5409 CBR0 711 (U. S. Army Waterway Experiment Station)

E (lb/in2) = 2550 CBR0 64 (Transport and Road Research Laboratory, England)

(See “Pavement Deflection Analysis,” FHWA Report HI-94-021, NHI, February 1994.) More detailed equations have been developed by correlating laboratory results with fundamental soil properties. R. F. Carmichael III and E. Stuart (“Predicting Resilient Modulus: A Study to Determine the Mechanical Properties of Subgrade Soils,” Transportation Research Record 1043, Transportation Research Board, National Research Council, Washington, D. C., 1985) developed the following models for the U. S. Forest Service:

Axle load				Slab thickness D, in
kips	kN	6	7	8	9	10	11	12	13	14
2	9	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001
4	18	0.0006	0.0006	0.0005	0.0005	0.0005	0.0005	0.0005	0.0005	0.0005
6	27	0.002	0.002	0.002	0.002	0.002	0.002	0.002	0.002	0.002
8	36	0.007	0.006	0.006	0.005	0.005	0.005	0.005	0.005	0.005
10	44	0.015	0.014	0.013	0.013	0.012	0.012	0.012	0.012	0.012
12	53	0.031	0.028	0.026	0.026	0.025	0.025	0.025	0.025	0.025
14	62	0.057	0.052	0.049	0.048	0.047	0.047	0.047	0.047	0.047
16	71	0.097	0.089	0.084	0.082	0.081	0.081	0.080	0.080	0.080
18	80	0.155	0.143	0.136	0.133	0.132	0.131	0.131	0.131	0.131
20	89	0.234	0.220	0.211	0.206	0.204	0.203	0.203	0.203	0.203
22	98	0.340	0.325	0.313	0.308	0.305	0.304	0.303	0.303	0.303
24	107	0.475	0.462	0.450	0.444	0.441	0.440	0.439	0.439	0.439
26	116	0.644	0.637	0.627	0.622	0.620	0.619	0.618	0.618	0.618
28	125	0.855	0.854	0.852	0.850	0.850	0.850	0.849	0.849	0.849
30	133	1.11	1.12	1.13	1.14	1.14	1.14	1.14	1.14	1.14
32	142	1.43	1.44	1.47	1.49	1.50	1.51	1.51	1.51	1.51
34	151	1.82	1.82	1.87	1.92	1.95	1.96	1.97	1.97	1.97
36	160	2.29	2.27	2.35	2.43	2.48	2.51	2.52	2.52	2.53
38	169	2.85	2.80	2.91	3.03	3.12	3.16	3.18	3.20	3.20
40	178	3.52	3.42	3.55	3.74	3.87	3.94	3.98	4.00	4.01
42	187	4.32	4.16	4.30	4.55	4.74	4.86	4.91	4.95	4.96
44	196	5.26	5.01	5.16	5.48	5.75	5.92	6.01	6.06	6.09
46	205	6.36	6.01	6.14	6.53	6.90	7.14	7.28	7.36	7.40
48	214	7.64	7.16	7.27	7.73	8.21	8.55	8.75	8.86	8.92
50	222	9.11	8.50	8.55	9.07	9.68	10.14	10.42	10.58	10.66
52	231	10.8	10.0	10.0	10.6	11.3	11.9	12.3	12.5	12.7
54	240	12.8	11.8	11.7	12.3	13.2	13.9	14.5	14.8	14.9
56	249	15.0	13.8	13.6	14.2	15.2	16.2	16.8	17.3	17.5
58	258	17.5	16.0	15.7	16.3	17.5	18.6	19.5	20.1	20.4
60	267	20.3	18.5	18.1	18.7	20.0	21.4	22.5	23.2	23.6
62	276	23.5	21.4	20.8	21.4	22.8	24.4	25.7	26.7	27.3
64	285	27.0	24.6	23.8	24.4	25.8	27.7	29.3	30.5	31.3
66	294	31.0	28.1	27.1	27.6	29.2	31.3	33.2	34.7	35.7
68	302	35.4	32.1	30.9	31.3	32.9	35.2	37.5	39.3	40.5
70	311	40.3	36.5	35.0	35.3	37.0	39.5	42.1	44.3	45.9
72	320	45.7	41.4	39.6	39.8	41.5	44.2	47.2	49.8	51.7
74	329	51.7	46.7	44.6	44.7	46.4	49.3	52.7	55.7	58.0
76	338	58.3	52.6	50.2	50.1	51.8	54.9	58.6	62.1	64.8
78	347	65.5	59.1	56.3	56.1	57.7	60.9	65.0	69.0	72.3
80	356	73.4	66.2	62.9	62.5	64.2	67.5	71.9	76.4	80.2
82	365	82.0	73.9	70.2	69.6	71.2	74.7	79.4	84.4	88.8
84	374	91.4	82.4	78.1	77.3	78.9	82.4	87.4	93.0	98.1
86	383	102.	92.	87.	86.	87.	91.	96.	102.	108.
88	391	113.	102.	96.	95.	96.	100.	105.	112.	119.
90	400	125.	112.	106.	105.	106.	110.	115.	123.	130.

Conversion: 1 in = 25.4 mm.

TABLE 3.15 Axle Load Equivalency Factors for Rigid Pavements, Triple Axles, and pt of 2.5 Axle load Slab thickness D, in kips kN 6 7 8 9 10 11 12 13 14 2 9 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 4 18 0.0003 0.0003 0.0003 0.0003 0.0003 0.0003 0.0003 0.0003 0.0003 6 27 0.001 0.001 0.001 0.001 0.001 0.001 0.001 0.001 0.001 8 36 0.003 0.002 0.002 0.002 0.002 0.002 0.002 0.002 0.002 10 44 0.006 0.005 0.005 0.005 0.005 0.005 0.005 0.005 0.005 12 53 0.011 0.010 0.010 0.009 0.009 0.009 0.009 0.009 0.009 14 62 0.020 0.018 0.017 0.017 0.016 0.016 0.016 0.016 0.016 16 71 0.033 0.030 0.029 0.028 0.027 0.027 0.027 0.027 0.027 18 80 0.053 0.048 0.045 0.044 0.044 0.043 0.043 0.043 0.043 20 89 0.080 0.073 0.069 0.067 0.066 0.066 0.066 0.066 0.066 22 98 0.116 0.107 0.101 0.099 0.098 0.097 0.097 0.097 0.097 24 107 0.163 0.151 0.144 0.141 0.139 0.139 0.138 0.138 0.138 26 116 0.222 0.209 0.200 0.195 0.194 0.193 0.192 0.192 0.192 28 125 0.295 0.281 0.271 0.265 0.263 0.262 0.262 0.262 0.262 30 133 0.384 0.371 0.359 0.354 0.351 0.350 0.349 0.349 0.349 32 142 0.490 0.480 0.468 0.463 0.460 0.459 0.458 0.458 0.458 34 151 0.616 0.609 0.601 0.596 0.594 0.593 0.592 0.592 0.592 36 160 0.765 0.762 0.759 0.757 0.756 0.755 0.755 0.755 0.755 38 169 0.939 0.941 0.946 0.948 0.950 0.951 0.951 0.951 0.951 40 178 1.14 1.15 1.16 1.17 1.18 1.18 1.18 1.18 1.18 42 187 1.38 1.38 1.41 1.44 1.45 1.46 1.46 1.46 1.46 44 196 1.65 1.65 1.70 1.74 1.77 1.78 1.78 1.78 1.79 46 205 1.97 1.96 2.03 2.09 2.13 2.15 2.16 2.16 2.16 48 214 2.34 2.31 2.40 2.49 2.55 2.58 2.59 2.60 2.60 50 222 2.76 2.71 2.81 2.94 3.02 3.07 3.09 3.10 3.11 52 231 3.24 3.15 3.27 3.44 3.56 3.62 3.66 3.68 3.68 54 240 3.79 3.66 3.79 4.00 4.16 4.26 4.30 4.33 4.34 56 249 4.41 4.23 4.37 4.63 4.84 4.97 5.03 5.07 5.09 58 258 5.12 4.87 5.00 5.32 5.59 5.79 5.85 5.90 5.93 60 267 5.91 5.59 5.71 6.08 6.42 6.64 6.77 6.84 6.87 62 276 6.80 6.39 6.50 6.91 7.33 7.62 7.79 7.88 7.93 64 285 7.79 7.29 7.37 7.82 8.33 8.70 8.92 9.04 9.11 66 294 8.90 8.28 8.33 8.83 9.42 9.88 10.17 10.33 10.42 68 302 10.1 9.4 9.4 9.9 10.6 11.2 11.5 11.7 11.9 70 311 11.5 10.6 10.6 11.1 11.9 12.6 13.0 13.3 13.5 72 320 13.0 12.0 11.8 12.4 13.3 14.1 14.7 15.0 15.2 74 329 14.6 13.5 13.2 13.8 14.8 15.8 16.5 16.9 17.1 76 338 16.5 15.1 14.8 15.4 16.5 17.6 18.4 18.9 19.2 78 347 18.5 16.9 16.5 17.1 18.2 19.5 20.5 21.1 21.5 80 356 20.6 18.8 18.3 18.9 20.2 21.6 22.7 23.5 24.0 82 365 23.0 21.0 20.3 20.9 22.2 23.8 25.2 26.1 26.7 84 374 25.6 23.3 22.5 23.1 24.5 26.2 27.8 28.9 29.6 86 383 28.4 25.8 24.9 25.4 26.9 28.8 30.5 31.9 32.8 88 391 31.5 28.6 27.5 27.9 29.4 31.5 33.5 35.1 36.1 90 400 34.8 31.5 30.3 30.7 32.2 34.4 36.7 38.5 39.8 Conversion: 1 in = 25.4 mm.

Cohesive soils:

MR = 37.431 – 0.4566(PI) – 0.6179(%W) – 0.1424(S200) +

0.1791(CS) – 0.3248(DS) + 36.422(CH) + 17.097(MH)

resilient modulus, kips/in2 plasticity index percentage water

percentage passing the no. 200 sieve confining stress, lb/in2 deviator stress, lb/in2

1 for CH soil (Unified Soil Classification, Art. 8.3.2)

0 otherwise

1 for MH soil (Unified Soil Classification)

0 otherwise

TABLE 3.17 Axle Load Equivalency Factors for Rigid Pavements, Tandem Axles, andpt of 3.0 Axle load Slab thickness D, in kips kN 6 7 8 9 10 11 12 13 14 2 9 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 0.0001 4 18 0.0007 0.0006 0.0005 0.0005 0.0005 0.0005 0.0005 0.0005 0.0005 6 27 0.003 0.002 0.002 0.002 0.002 0.002 0.002 0.002 0.002 8 36 0.008 0.006 0.006 0.006 0.005 0.005 0.005 0.005 0.005 10 44 0.018 0.015 0.013 0.013 0.013 0.012 0.012 0.012 0.012 12 53 0.036 0.030 0.027 0.026 0.026 0.025 0.025 0.025 0.025 14 62 0.066 0.056 0.050 0.048 0.047 0.047 0.047 0.047 0.047 16 71 0.111 0.095 0.087 0.083 0.081 0.081 0.081 0.080 0.080 18 80 0.174 0.153 0.140 0.135 0.132 0.131 0.131 0.131 0.131 20 89 0.260 0.234 0.217 0.209 0.205 0.204 0.203 0.203 0.203 22 98 0.368 0.341 0.321 0.311 0.307 0.305 0.304 0.303 0.303 24 107 0.502 0.479 0.458 0.447 0.443 0.440 0.440 0.439 0.439 26 116 0.664 0.651 0.634 0.625 0.621 0.619 0.618 0.618 0.618 28 125 0.859 0.857 0.853 0.851 0.850 0.850 0.850 0.849 0.849 30 133 1.09 1.10 1.12 1.13 1.14 1.14 1.14 1.14 1.14 32 142 1.38 1.38 1.44 1.47 1.49 1.50 1.51 1.51 1.51 34 151 1.72 1.71 1.80 1.88 1.93 1.95 1.96 1.97 1.97 36 160 2.13 2.10 2.23 2.36 2.45 2.49 2.51 2.52 2.52 38 169 2.62 2.54 2.71 2.92 3.06 3.13 3.17 3.19 3.20 40 178 3.21 3.05 3.26 3.55 3.76 3.89 3.95 3.98 4.00 42 187 3.90 3.65 3.87 4.26 4.58 4.77 4.87 4.92 4.95 44 196 4.72 4.35 4.57 5.06 5.50 5.78 5.94 6.02 6.06 46 205 5.68 5.16 5.36 5.95 6.54 6.94 7.17 7.29 7.36 48 214 6.80 6.10 6.25 6.93 7.69 8.24 8.57 8.76 8.86 50 222 8.09 7.17 7.26 8.03 8.96 9.70 10.17 10.43 10.58 52 231 9.57 8.41 8.40 9.24 10.36 11.32 11.96 12.33 12.54 54 240 1.13 9.8 9.7 10.6 11.9 13.1 14.0 14.5 14.8 56 249 13.2 11.4 11.2 12.1 13.6 15.1 16.2 16.9 17.3 58 258 15.4 13.2 12.8 13.7 15.4 17.2 18.6 19.5 20.1 60 267 17.9 15.3 14.7 15.6 17.4 19.5 21.3 22.5 23.2 62 276 20.6 17.6 16.8 17.6 19.6 22.0 24.1 25.7 26.6 64 285 23.7 20.2 19.1 19.9 22.0 24.7 27.3 29.2 30.4 66 294 27.2 23.1 21.7 22.4 24.6 27.6 30.6 33.0 34.6 68 302 31.1 26.3 24.6 25.2 27.4 30.8 34.3 37.1 39.2 70 311 35.4 29.8 27.8 28.2 30.6 34.2 38.2 41.6 44.1 72 320 40.1 33.8 31.3 31.6 34.0 37.9 42.3 46.4 49.4 74 329 45.3 38.1 35.2 35.4 37.7 41.8 46.8 51.5 55.2 76 338 51.1 42.9 39.5 39.5 41.8 46.1 51.5 56.9 61.3 78 347 57.4 48.2 44.3 44.0 46.3 50.7 56.6 62.7 67.9 80 356 64.3 53.9 49.4 48.9 51.1 55.8 62.1 68.9 74.9 82 365 71.8 60.2 55.1 54.3 56.5 61.2 67.9 75.5 82.4 84 374 80.0 67.0 61.2 60.2 62.2 67.0 74.2 82.4 90.3 86 383 89.0 74.5 67.9 66.5 68.5 73.4 80.8 89.8 98.7 88 391 98.7 82.5 75.2 73.5 75.3 80.2 88.0 97.7 107.5 90 400 109. 91. 83. 81. 83. 88. 96. 106. 117 Conversion: 1 in = 25.4 mm.

Axle load				Slab thickness D, in
kips	kN	6	7	8	9	10	11	12	13	14
2	9	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001	0.0001
4	18	0.0004	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003	0.0003
6	27	0.001	0.001	0.001	0.001	0.001	0.001	0.001	0.001	0.001
8	36	0.003	0.003	0.002	0.002	0.002	0.002	0.002	0.002	0.002
10	44	0.007	0.006	0.005	0.005	0.005	0.005	0.005	0.005	0.005
12	53	0.013	0.011	0.010	0.009	0.009	0.009	0.009	0.009	0.009
14	62	0.023	0.020	0.018	0.017	0.017	0.016	0.016	0.016	0.016
16	71	0.039	0.033	0.030	0.028	0.028	0.027	0.027	0.027	0.027
18	80	0.061	0.052	0.047	0.045	0.044	0.044	0.043	0.043	0.043
20	89	0.091	0.078	0.071	0.068	0.067	0.066	0.066	0.066	0.066
22	98	0.132	0.114	0.104	0.100	0.098	0.097	0.097	0.097	0.097
24	107	0.183	0.161	0.148	0.143	0.140	0.139	0.139	0.138	0.138
26	116	0.246	0.221	0.205	0.198	0.195	0.193	0.193	0.192	0.192
28	125	0.322	0.296	0.277	0.268	0.265	0.263	0.262	0.262	0.262
30	133	0.411	0.387	0.367	0.357	0.353	0.351	0.350	0.349	0.349
32	142	0.515	0.495	0.476	0.466	0.462	0.460	0.459	0.458	0.458
34	151	0.634	0.622	0.607	0.599	0.595	0.594	0.593	0.592	0.592
36	160	0.772	0.768	0.762	0.758	0.756	0.756	0.755	0.755	0.755
38	169	0.930	0.934	0.942	0.947	0.949	0.950	0.951	0.951	0.951
40	178	1.11	1.12	1.15	1.17	1.18	1.18	1.18	1.18	1.18
42	187	1.32	1.33	1.38	1.42	1.44	1.45	1.46	1.46	1.46
44	196	1.56	1.56	1.64	1.71	1.75	1.77	1.78	1.78	1.78
46	205	1.84	1.83	1.94	2.04	2.10	2.14	2.15	2.16	2.16
48	214	2.16	2.12	2.26	2.41	2.51	2.56	2.58	2.59	2.60
50	222	2.53	2.45	2.61	2.82	2.96	3.03	3.07	3.09	3.10
52	231	2.95	2.82	3.01	3.27	3.47	3.58	3.63	3.66	3.68
54	240	3.43	3.23	3.43	3.77	4.03	4.18	4.27	4.31	4.33
56	249	3.98	3.70	3.90	4.31	4.65	4.86	4.98	5.04	5.07
58	258	4.59	4.22	4.42	4.90	5.34	5.62	5.78	5.86	5.90
60	267	5.28	4.80	4.99	5.54	6.08	6.45	6.66	6.78	6.84
62	276	6.06	5.45	5.61	6.23	6.89	7.36	7.64	7.80	7.88
64	285	6.92	6.18	6.29	6.98	7.76	8.36	8.72	8.93	9.04
66	294	7.89	6.98	7.05	7.78	8.70	9.44	9.91	10.18	10.33
68	302	8.96	7.88	7.87	8.66	9.71	10.61	11.20	11.55	11.75
70	311	10.2	8.9	8.8	9.6	10.8	11.9	12.6	13.1	13.3
72	320	11.5	10.0	9.8	10.6	12.0	13.2	14.1	14.7	15.0
74	329	12.9	11.2	10.9	11.7	13.2	14.7	15.8	16.5	16.9
76	338	14.5	12.5	12.1	12.9	14.5	16.2	17.5	18.4	18.9
78	347	16.2	13.9	13.4	14.2	15.9	17.8	19.4	20.5	21.1
80	356	18.2	15.5	14.8	15.6	17.4	19.6	21.4	22.7	23.5
82	365	20.2	17.2	16.4	17.2	19.1	21.4	23.5	25.1	26.1
84	374	22.5	19.1	18.1	18.8	20.8	23.4	25.8	27.6	28.8
86	383	25.0	21.2	19.9	20.6	22.6	25.5	28.2	30.4	31.8
88	391	27.6	23.4	21.9	22.5	24.6	27.7	30.7	33.2	35.0
90	400	30.5	25.8	24.1	24.6	26.8	30.0	33.4	36.3	38.3

Conversion: 1 in = 25.4 mm.

Analysis period

years

Location

Assumed SN or D =____________ in

Granular soils:

(3.6)

Analysis period =_____ 20____ years

Assumed SN or D =_____ 9_____ in

	Current	Growth	Design	ESAL	Design
Vehicle types	traffic (A)	factors (B)	traffic (C)	factor (D)	ESAL (E)
		2%
Passenger cars	5,925	24.30	52,551,787	0.0008	42,041
Buses	35	24.30	310,433	0.6806	211,280
Panel and pickup trucks	1,135	24.30	10,066,882	0.0122	122,816
Other 2-axle/4-tire trucks	3	24.30	26,609	0.0052	138
2-axle/6-tire trucks	372	24.30	3,299,454	0.1890	623,597
3 or more axle trucks All single-unit trucks	34	24.30	301,563	0.1303	39,294
3-axle tractor semitrailers	19	24.30	168,521	0.8646	145,703
4-axle tractor semitrailers	49	24.30	434,606	0.6560	285,101
5+ axle tractor semitrailers All tractor semitrailers	1,880	24.30	16,674,660	2.3719	39,550,626
5-axle double trailers	103	24.30	913,559	2.3187	2,118,268
6+ axle double trailers All double trailer combos	0	24.30
3-axle truck-trailers	208	24.30	1,844,856	0.0152	28,042
4-axle truck-trailers	305	24.30	2,705,198	0.0152	41,119
5+ axle truck-trailers All truck-trailer combos	125	24.30	1,108,688	0.5317	589,489
All vehicles	10,193		90,406,816	Design ESAL	43,772,314

Location

Example 1

Source: Guide for Design of Pavement Structures, American Association of State Highway and Transportation Officials, Washington, D. C., 1993, with permission.

TABLE 3.21 Equivalency Factors for Determining ESAL Rigid pavement Flexible pavement Function classification B C B C Rural interstate 2.27 0.914 1.60 0.735 All other rural 2.16 1.02 1.44 0.777 Urban interstate, freeway, and expressway 2.50 1.48 1.74 1.13 All other urban 1.61 0.673 1.11 0.534 Notes: B = tractor-trucks with semitrailers and trucks with trailers. C = single-unit trucks (2 axles, 6 tires or more). Source: Ohio Department of Transportation, Location and Design Manual, Vol. 1, Roadway Design, December 1990, revised October 1992, with permission.

REPEATED LOAD ACTUATOR

:SOLID BASES

SECTION VIEW

Note: LVDT tips shall rest on the triaxial cell itself or on a

plate/bracket which is rigidly attached to the tnaxial cell

FIGURE 3.9 Triaxial test chamber for determining resilient modulus of soil specimen. (From NRC Operational Guide No. SHRP-LTPP-OG-004, “SHRP-LTPP Interim Guide for Laboratory Material Handling and Testing," with permission)